6
$\begingroup$

I would like to calibrate Hull-White model using volatility data.I am using [Park (2004)] paper as a reference.

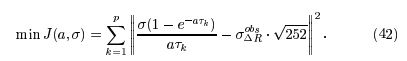
He suggests to minimize the following objective function: enter image description here

where the first term is theoretical (H-W) conditional volatility [st. dev.] of changes of the spot rates and the second term is defined as:

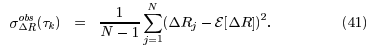enter image description here which is sample variance of observed market data.

My question is:

  • why do we subtract variance from volatility[standard deviation] in the objective function? (i.e. not variance - variance).

NOTE: Initially, I thought this was a mistake, but the same expression is used for the two factor model as well (formula (158) in the paper). In addition, I tried to calibrate the model using both (standard deviation - variance) and (standard deviation - standard-deviation) approaches. It seems like the results from (standard deviation-variance) case, as in Park(2004), make more sense.

Thank You

Improve this question
$\endgroup$
1
  • $\begingroup$ could you elaborate on what you mean by "make more sense" ? $\endgroup$
    – Probilitator
    Commented Feb 18, 2014 at 10:19

1 Answer 1

Reset to default
3
$\begingroup$

I took a look at the paper and would contend that it is a typo. I would assume he just copy-pasted the equation - for it is exactly the same for the two factor model cf. eq (157) and eq (41)

If you follow his reasoning and his notation it would make no sense to use the observed sample variance. He always denotes the variace by $\sigma^2$ and the standard-deviation by $\sigma$ or $\sigma(t)$

Also, it would make no sense to compare standard deviation to variance - your objective function would be not very sensitive to changes in the observed variances. For variances being the squared number of a $\sigma <1$ will always be much smaller. Also you objective function would not evaluate the case $\sigma = \sigma^{obs}$ properly, with $\sigma > (\sigma^{obs})^2$.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thanks. Finally, I have also concluded that it was a typo. But I have one more question. Is it possible to measure if the calibration was significant ? $\endgroup$
    – Mstudent1
    Commented Mar 13, 2014 at 2:49
  • $\begingroup$ what exactly do you mean by significant ? a criteria to decide whether the calibration is any good ? $\endgroup$
    – Probilitator
    Commented Mar 13, 2014 at 15:39
  • $\begingroup$ Yes. Actually, the most natural way to do this is to compare the observed volatility and Hull-White implied volatility in a volatility-maturity space. But is there any rule which would allow to conclude that the calibration is successful (or significant), i.e. the difference between volatilities is small enough? $\endgroup$
    – Mstudent1
    Commented Mar 14, 2014 at 9:01
  • $\begingroup$ it depends on what you are pricing. In most cases a calibration is considered to work well if it fits the most relevant vols or prices best. If you were pricing a cap with short maturity you would want to hit the short term implied vols best. $\endgroup$
    – Probilitator
    Commented Mar 14, 2014 at 9:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.