Consider reading Lorenzo Bergomi's excellent book -- or at least the first chapter available here for download --, it will help you clarify things.
Some remarks as to your original question:
- It is well known that, under a pure diffusion assumption, the total P&L of a delta hedged European option (i.e. an option whose payoff only depends on the value of the underlying asset at a future date $T$) over the horizon $[0,T]$ writes:
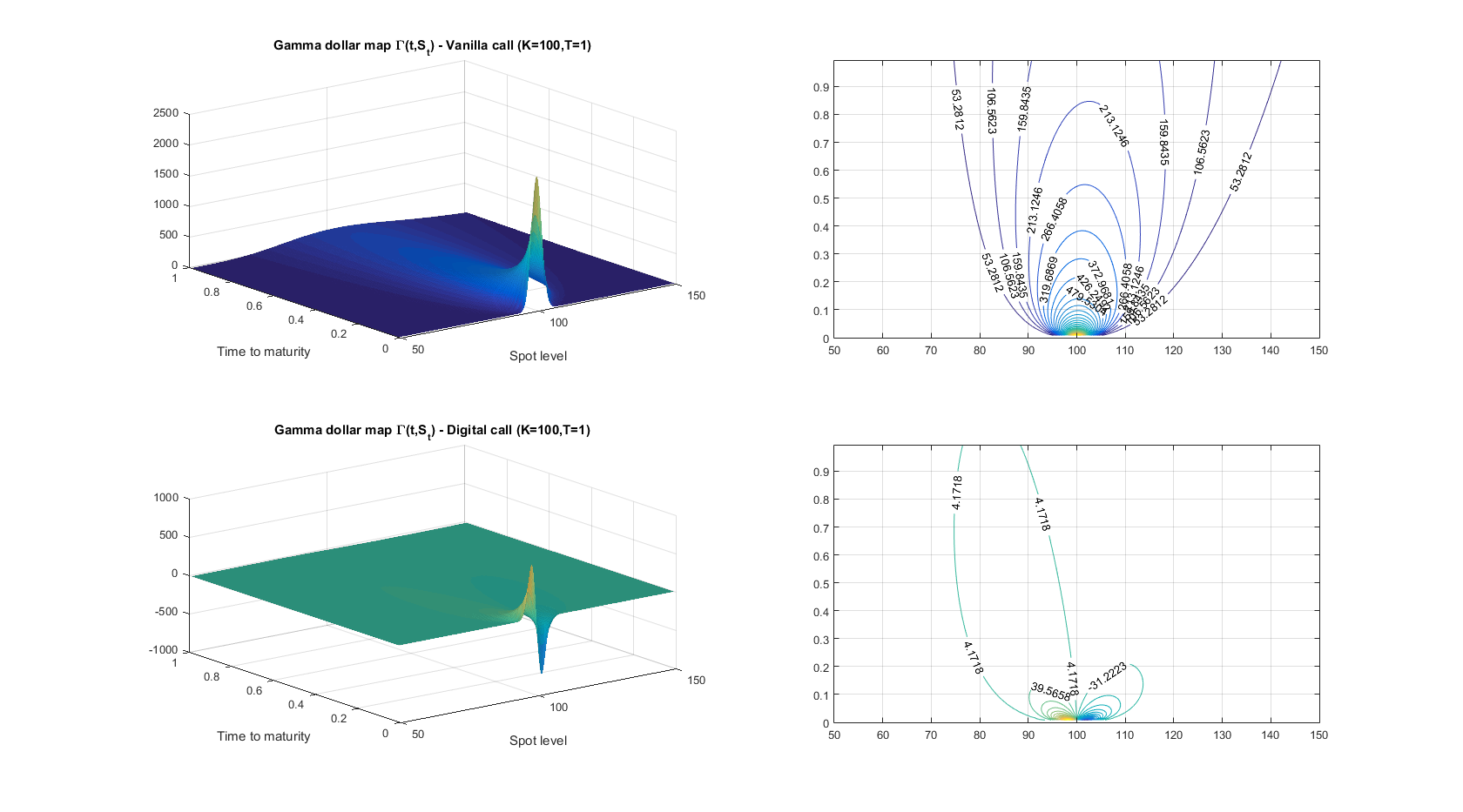
$$ P\&L_{[0,T]} = \int_0^T \frac{1}{2} \underbrace{\Gamma(t,S_t,\sigma^2_{t,\text{impl.}})S_t^2}_{\text{Gamma dollar}}( \sigma^2_{t,\text{real.}} - \sigma^2_{t,\text{impl.}}) dt $$
As such, although a delta hedged European option portfolio is sensitive to the realised vs. implied volatility discrepancy, it is not a pure volatility trade: the Gamma dollar term introduces a dependency on the spot path. This Gamma dollar term should really be seen as some kind of volatility accumulator: only along paths where the Gamma dollar is non zero will the discrepancy between realised and implied volatility matter.
- The previous relationship still holds for digital (or binary) options, since they are also European options after all. What you observe is thus simply a consequence of the fact that the Gamma map of a binary call is pretty different from that of a vanilla call (see below): indeed, it is zero over most of the time to maturity/spot domain. As such the contribution of realised vs. implied volatility discrepancies to the total P&L is completely different: only what happens (1) around the strike, (2) near the expiry matters. This is why you did not observe a strong correlation between the resulting P&L and the realised vol over the entire delta-hedging horizon.
Now with respect to delta hedging exotic options, quoting from Lorenzo Bergomi's "chapter's digest" and adding some of my own remarks:
Delta hedging removes the order-one contribution of $\delta S$ to the P&L of an option position.
Although the expectation of the P&L of a delta hedged portfolio is zero (provided you correctly anticipate the volatility - or variance - of future returns),
Delta hedging is not adequate for reducing the standard deviation of the P&L [...] The sources of the dispersion of this P&L are: (a) the tails of returns, (b) the volatility of realized volatility and the correlation of future realized volatilities.
Using options for gamma-hedging (...)
In other words, using other vanilla options to locally cancel the gamma dollar term,
immunizes us against realized volatility (...) Dynamical trading of vanilla options, however, exposes us to uncertainty as to future levels of implied volatilities.
Hence the need of stochastic volatility models for modelling the dynamics of implied volatility.
Exotic options often depend in a complex way on the dynamics of implied volatilities
An exposure which is usually dealt with by trading vanilla options. See for instance the case of a (relative) forward-start at the money call in a homogeneous diffusion model. In that very specific case, we do not care about delta hedging anymore since it is only the dynamics of forward volatility that matters. One way want to neutralise such an exposure using calendar spreads.

