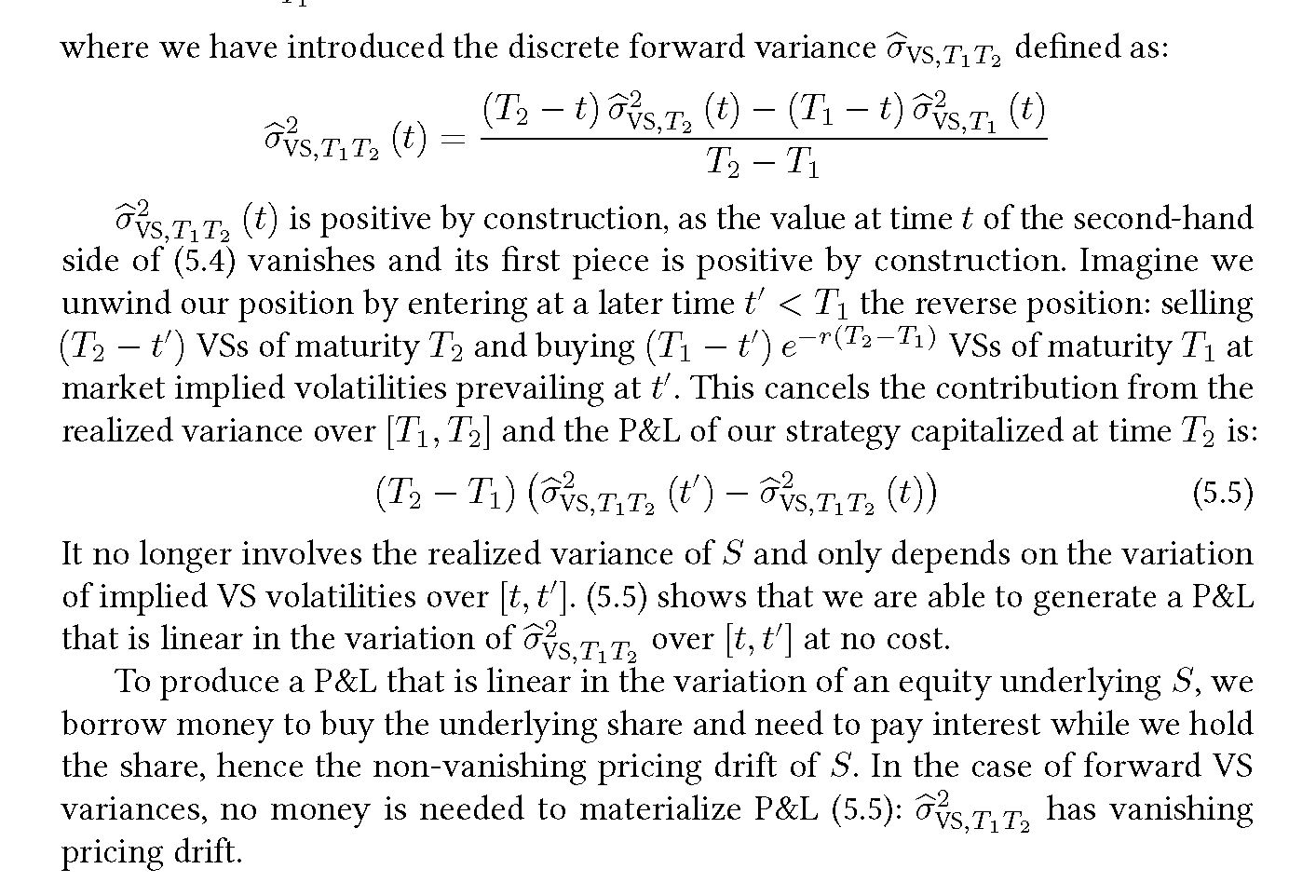
Short answer
He's basically making a parallel between a forward variance trade and a futures trade. In both cases you should have that the underlying quotes are martingales in the absence of arbitrage.
Long(er) answer
Under the physical measure $\Bbb{P}$, an arbitrage is a (self-financing) trading strategy $V$ - or rather the value of a portfolio implementing this strategy - for which there exists a time $T > 0$ such that
$$ V_0=0,\,\, V_T \geq 0\,\, \Bbb{P}-\text{a.s. and } \Bbb{P}(V_T \ne 0) > 0$$
Suppose you define an equivalent probability measure $\Bbb{Q}\equiv\Bbb{P}$. Since by definition, both measures agree on null events, our arbitrage definition translates to
$$ V_0=0,\,\, V_T \geq 0\,\, \Bbb{Q}-\text{a.s. and } \Bbb{Q}(V_T \ne 0) > 0 \tag{A}$$
Notice that if $\Bbb{Q}$ is further a martingale measure, that is if $(V_t)_{t\geq0}$ emerges as a $\Bbb{Q}$-martingale:
$$ V_0 = \Bbb{E}_0^\Bbb{Q} [ V_T ] $$
then $(A)$ will never happen. This explains the central role of equivalent martingale measures in arbitrage pricing theory.
Putting that back into context, you've managed to identify a (self-financing) strategy (i.e. buying and selling forward variance swaps), which at no cost ($V_t=0$), allows you to earn a quantity $$V_{t'} = (T_2-T_1) \left( \hat{\sigma}_{VS,T_1T_2}^2(t') - \hat{\sigma}_{VS,T_1T_2}^2(t)\right)$$
Based on what we've said earlier, in the absence of arbitrage, there should exist a measure $\Bbb{Q} \equiv \Bbb{P}$ such that
$$ \Bbb{E}^\Bbb{Q}_{t}[V_{t'}] = V_t$$
hence, using the definitions of $V_t$ and $V_{t'}$,
$$ \Bbb{E}^\Bbb{Q}_{t}\left[ \hat{\sigma}_{VS,T_1T_2}^2(t') \right] = \hat{\sigma}_{VS,T_1T_2}^2(t) $$
hence forward variance swap quotes are martingales. Assuming a continuous paths process (= in a diffusive setting), by the martingale representation theorem we should then have
$$ \hat{\sigma}_{VS,T_1T_2}^2(t) = ... dW_t^\Bbb{Q} $$
hence no pricing drift under $\Bbb{Q}$.