Delta-hedging frequency directly affects PnL, and not just PnL smoothness and variance?

Question

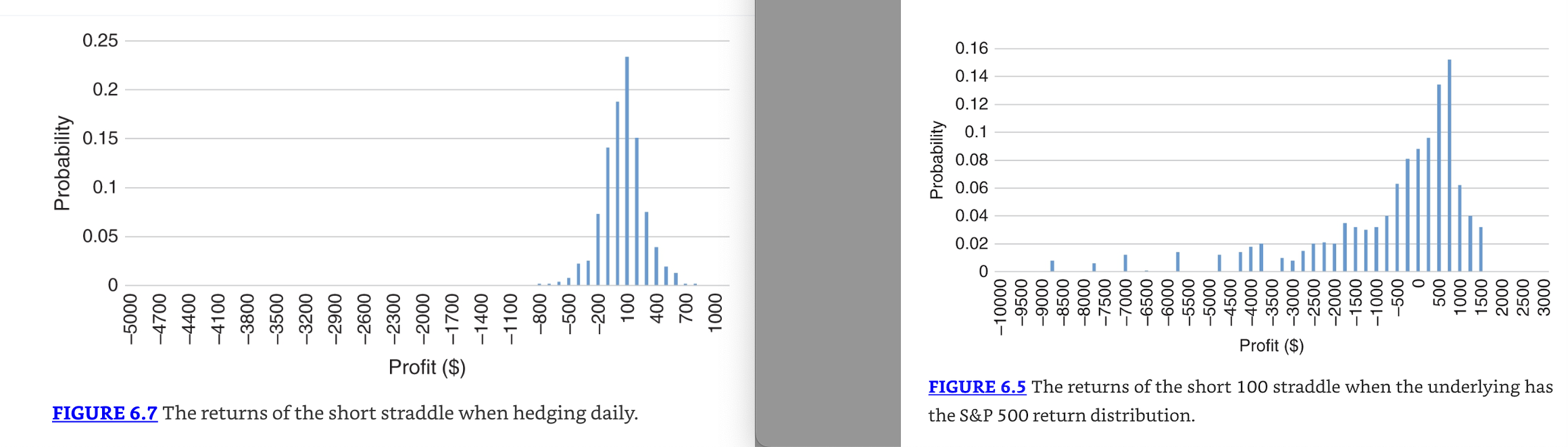
The information I have found about delta hedging frequency and (gamma) PnL on this site and numerous others all reiterate the same thing: that the frequency at which you delta-hedge only has an effect on the smoothness and variance of your PnL.

Nivel Egres: From the perspective of gamma pnl, the only thing that matters is the change in your asset price. Frequency is irrelevant - you can rebalance at different time periods or when delta exceeds a threshold or many other things - it is still an approximation of continuous integral and your expected P&L would be the same. For example, in real life, you are usually trying to optimize your hedging for a balance of (a) smoothness of P&L, (b) transaction costs, (c) vol dampening or amplifying (d) mean reversion found in the asset you're trading and (e) your risk limits.

How is this true though? Delta-hedging frequency has a direct effect on your PnL, and not just the smoothness of it.

So the thought here is that a trader who delta-hedges every minute, and a trader who hedges every end of day at market close, will both have the same expected profit at option expiry and only their PnL smoothness/variance will differ. Let's put this to the test.

We'll use this hypothetical scenario. And for the sake of simplicity we ignore transaction costs:

Two traders have bought a 100 strike ATM straddle (long gamma) that expires in a week on stock XYZ. The stock price is 100. They are both initially delta neutral. Throughout expiry, Trader A delta-hedges every minute, and trader B hedges every end of day at market close.

At 2:00 PM, stock XYZ drops -2% to 98. Trader A is delta-hedging the straddle every minute anyways so he buys shares to hedge and become delta-neutral again. The stock doesn't move at all until at 2:05 PM stock XYZ shoots up 4% from 98 to 101.92. Trader A sells the shares he previously bought, and then short sells some more shares to become delta-neutral. He has effectively locked in his gamma scalping PnL. The stock doesn't move at all again until 3:59 PM where the stock drops -1.88% from 101.92 to exactly 100. Trader A buys back his short shares, locks in his gamma PnL again, and is completely delta neutral.

Meanwhile it's the end of the day and time for Trader B to hedge, but he has nothing to delta-hedge because the stock is 100 at the end of the trading day, the same price at which he bought the ATM straddle and his delta of the position is 0.

Trader A has made some hefty PnL, meanwhile Trader B comes out with nothing at all and his missed out on volatility during the trading day which he could've profited off of had he been continuously hedging instead of just once a day.

So how does delta-hedging frequency just affect the smoothness and variance of PnL if we can clearly see it affects PnL itself in this example?

Answer 1

If you look at just a single example, it may seem like the frequency of hedging directly effects the EV/Avg(Pnl), like in the situation you described where hedging every minute proved to be more profitable. But you need to think about the question in a bigger picture sense. How would hedging frequency affect the results over thousands of simulations?

If you hedge every minute, you wouldn't realize the full pnl of the larger SD moves but you do capture the full pnl of the smaller intraday moves. Conversely, if you only hedge once per day, you won't realize the full pnl from the smaller intraday moves (like in your example) but you would in return realize the full pnl from the larger SD moves. Infrequent larger gains from larger SD moves balance out against the more frequent missed gains from smaller intraday moves in EV/Avg(PnL). [Sinclair does a great job explaining this in his books, pictures below provided].

At the end of the day, the EV/Avg(PNL) boils down to iv vs rv of stock. If those two are equal, then the EV/PNL will be the same for both traders regardless of hedging frequency. The only difference will be the variance of their PNL as described above.

Food for thought, the below example is just in theory and doesn't exist:

Now, in the above explanation, we assumed the stock was performing on some constant vol at all moments in time. What if the intraday vol diverges significantly from the daily vol? Ie: As an EXAGGERATION, say you look at some stock and you calculate from the past 10 day closing prices that the stock is performing on a 1 vol. Pretty much closes where it opened each day. You then decide to look closer and measure vol in 30 minute increments rather than by daily closing prices. When you look intraday/30 min increments, you see the stock moves a lot, but based on closing prices performs still on a 1 vol. In this case, when we measure vol in smaller 30 min increments, we can see it is significantly different than vol measured on close to close prices. Both traders buy the straddle on a 1 vol let's say, who do you think would be better off? The person who hedges several times a day or the person who hedges once at the end of the day? In this case, the stock is not performing at some constant vol at all moments in time over the duration of the life of the option and throughout each day, instead we can see the intraday vol is significantly different that the daily close to close vol.

In theory, if the above example existed, I WOULD THINK this would imply that hedging more frequently would be more profitable. HOWEVER, THIS ALSO WOULD IMPLY THAT THERE IS SOME PROFITABLE OUTRIGHT STOCK TRADING STRATEGY, WHICH WOULD IMPLY WE KNOW WHICH DIRECTION THE STOCK IS GOING TO GO NEXT, WHICH IN REALITY WE DON'T KNOW. Ie: If we know the stock is going to close near the opening price because it always performs on a 1 vol, and its noon and the stock is down -10%, we know that it has to go higher in the last few hours of the day and we could just outright buy stock to make money.

Answer 2

Under the assumptions of GBM - namely that periodic returns are independent of one another - then hedging frequency will have 0 impact on the expected P/L over time. If there is autocorrelation in the intraday return process that you choose to hedge at (which will in turn affect daily annualised volatility), then your P/L is definitely affected by your choice of hedging interval.

However, the existence of significant autocorrelation in the return process would hint that we are able to trade using futures/linear products on a intraday horizon which would probably (after accounting for liquidity and theta) prove more profitable to trade than the delta hedging strategy.

Over any longer period of time, there is not often a statistically significant autocorrelation in high frequency returns. If there was, then the above would be applicable which would dampen the effect.

The net effect of all that is that increased delta hedging frequency does just have the smoothing effect on P/L over long enough time horizons. But like you indicate you are exposed to one-off or rare mean reversion (or trend) effects, but these dissipate over large samples.