In the case of a vanilla bond I know that the duration will be less than the time to maturity.
But I am observing that for a non-vanilla bond, the duration is greater than time to maturity.
Can somebody provide the intuition for this? How is this possible and why this is the case?
Like Aksakal already mentioned in his comment it might depend on the duration formula you use. (see e.g. the wikipedia page or here) It can also depend on the type of instrument as mentioned by Richard.
This topic has also been already discussed on the Wilmott Forum (their proposed solution is a reverse floater)
Theoretically bonds with embedded options (e.g. callable bonds) could also produce a duration greater than their maturity. For such instruments a different approach is needed to calculate their duration.(see effective duration - you will have to scroll down a bit )
$Duration_{eff}=\frac {V_{-\Delta y}-V_{+\Delta y}}{2(V_0)\Delta y}$
Thus if there was a strong assymmetry in the sensitivity to up and down movements of the interest rate the effective duration could produce large and even negative values.
Note: the effective duration is the weighted sum of directional derivatives. The resulting value no longer has an interpreation as a point in time. It is primarily a price sensitivity measure.
Another approach to reproducing a negative duration is to use the "break-even"-interpretation
Some interpret duration as the "break even point" or length of time needed to hold a security to break even. A 100 bond that sells for 110 needs extra dividend payments to break even. Thus: the higher the earlier cashflows the lower the duration.
Thus most straight forward case to reproduce a duration greater than time to maturity is adding a negative cash-flow - this way we postpone the "break even point" (I must admit - the resulting product will no longer be a stereotypical bond)
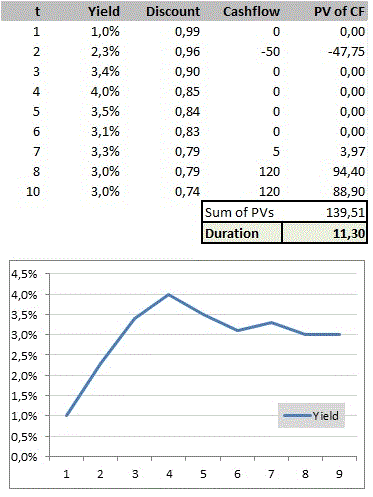
Using the Macaulay duration and assuming that the discount factors are given by $e^{-t*Yield_t}$ I have created a simple example (see table below)
In the example below we have an extreme case: negative cash-flow quite early and the offsetting cash-flows are only due at the end of maturity. Thus the formula outputs a duration greater than time to maturity Indicating that the "break-even" happens very late.
Note: The interpretation of duration as the "break-even-time" has only an indicational value. Thus it is often not the actual break-even-time but more of an indicator of how long you might have to wait until you have rcouperated your investment.
In Bonds with a negative yield the duration should be longer than the maturity.
Duration is the length of time for the return of the fund.
As long as the coupons are positive, the investor returns the fund before the final redemption, in a negative interest rate situation the negative interest rate eats from the fund and the duration should be longer than the maturity.
