11
$\begingroup$

Where can I find more VaR and CVaR formulas for continuous distributions?

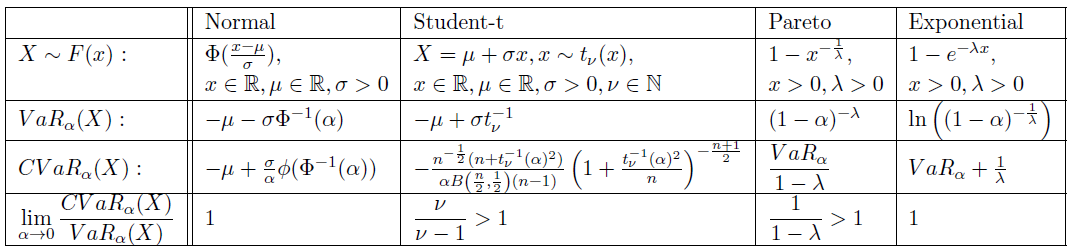
I collected a list here: enter image description here

Improve this question
$\endgroup$

3 Answers 3

Reset to default
9
$\begingroup$

Values of VaR are just the inverses of the cumulative distributions.

CVaR is not a very commonly used term, its more frequently used synonym is Expected Shortfall. See http://www.maths.manchester.ac.uk/~saralees/chap17.pdf for the list of Expected Shortfall values for more than 20 distributions.

Improve this answer
$\endgroup$
7
  • $\begingroup$ I am looking for a list of $VaR$ and $CVaR$ together, because the notation for the distribution function differs sometimes? $\endgroup$
    – emcor
    Commented Oct 22, 2014 at 14:57
  • $\begingroup$ @emcor: in the doc I have referenced, the author gives you the CDFs before deriving ESs. VaR = inverse of CDF! $\endgroup$
    – Yulia V
    Commented Oct 22, 2014 at 15:04
  • $\begingroup$ I doubt that you can find any of the 20 inverses there yourself, except for maybe normal distribution. So its useless to give the cdf without inverse. $\endgroup$
    – emcor
    Commented Oct 22, 2014 at 15:15
  • 1
    $\begingroup$ I disagree with your assertion that CVaR is not a commonly used term. They are interchangeable, as far as I'm concerned. $\endgroup$
    – John
    Commented Oct 22, 2014 at 17:15
  • 1
    $\begingroup$ Great link I think. +1 $\endgroup$
    – SRKX
    Commented Oct 23, 2014 at 4:04
3
$\begingroup$

More often than not, I prefer to work with a scenario representation. That is, I will simulate from the distribution and calculate the VaR and CVaR as appropriate. This is especially the case for forward-looking analysis of portfolios' CVaR, rather than in evaluating the historical returns of some portfolio.

If for some reason I can't do the scenario approach, then I will use the Cornish-Fisher approximation. There is a paper by Boudt, Peterson, and Croux that I think provides the formula for both VaR and CVaR, as well as a few others (perhaps refer to other references, and there's already a question on this site about it wrt VaR). If you're using Cornish-Fisher, then you can write the VaR and CVaR in terms of the moments of whatever distribution you're looking at. BPC provides the formula for portfolios as well, but in my experience this is a big pain.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thanks for your insight. However, I was looking for some exact formulas of VaR, CVaR for different parametric distributions just for research purposes... $\endgroup$
    – emcor
    Commented Oct 22, 2014 at 19:37
  • 1
    $\begingroup$ Other than the one posted by @YuliaV, I'm not aware of any papers like that off the top of my head. In practice, I just don't use the analytic formula often (really only for normal VaR). Not sure how common that is for others. $\endgroup$
    – John
    Commented Oct 22, 2014 at 20:19
  • $\begingroup$ @John, could you please give a reference on scenario approach? $\endgroup$
    – Nick
    Commented Nov 17, 2016 at 6:55
  • 1
    $\begingroup$ @Nick The original work by Rockafellar and Urysev describes the scenario approach. See equation 17 of ise.ufl.edu/uryasev/files/2011/11/CVaR1_JOR.pdf $\endgroup$
    – John
    Commented Nov 18, 2016 at 21:18
2
$\begingroup$

The paper "Calculating CVaR and bPOE for Common Probability Distributions With Application to Portfolio Optimization and Density Estimation" by Norton, Matthew; Khokhlov, Valentyn; Uryasev, Stan (2018) gives a large number of CVAR analytical formula with full proof.

Most of them can also be found on the Expected shortfall (aka CVAR) Wikipedia page.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.