0
$\begingroup$

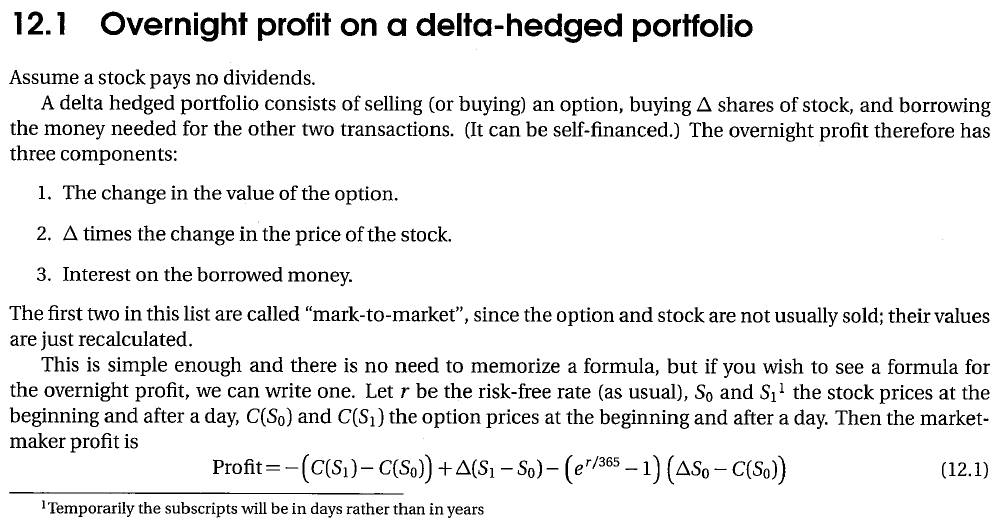
I am self-studying and encountered the following passage from my textbook on the market maker's overnight profit on a delta-hedged portfolio:

enter image description here

I don't understand why their isn't a factor of $(e^{r/365} - 1)$ multiplied by the $C(S_0)$ term. My reasoning is that if a call/put is sold, the positive cash flow from the premium could be invested at the risk-free rate.

My understanding is that the overnight profit on a sold call would be the premium that the call sold for at day 0, which could be invested at the risk free rate overnight, minus the premium that the call could be sold for if held on to for another day, or $C(S_0)\left(e^{r/365} - 1\right) - C(S_1)$

Wouldn't it be more accurate to say that: $$\text{Profit} = -\left(C(S_1) - C(S_0)(e^{r/365} - 1)\right) +\Delta(S_1 - S_0) - (e^{r/365} - 1)\left(\Delta S_0 - C(S_0)\right)?$$

If we're factoring interest lost on self-financing the selling of an option and buying $\Delta$ shares of stock, why wouldn't we also factor interest gained by selling the option at $T = 0$?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ There is already an interest term in formula 12.1 on the net of the amount needed to buy the stock minus the cash proceeds from the option. So the interest from investing the cash from the option is already taken into account. The sign of the interest term will depend on whether $\Delta S_o$ or $C(S_0)$ is bigger. You either pay or receive interest according to your net borrowing/lending. $\endgroup$
    – nbbo2
    Commented Nov 18, 2016 at 17:04

1 Answer 1

Reset to default
1
$\begingroup$

If you keep a delta-hedged portfolio $\pi$, your position looks like this:

$\pi=-C+\Delta S$

and it is worth $\pi$. If you get more money for selling call option that is needed for buying underlying to hedge, you invest it in the risk free money market account. If the money proceeds from selling the call option are not enough to buy the underlying, you borrow from the money market account at the risk free rate.

If it is hedged it has no risk, so it should earn the risk free rate, so overnight you earn what portfolio was worth the day before times risk free rate: $\pi (e^{r/365}-1)=(-C+\Delta S)(e^{r/365}-1)$

Equation from the reading (with extra change in the price of option and stock) assumes that your portfolio was not delta-neutral and the hedging was not effective - this happens when the moves in the underlying are large overnight. So effectively the equation from the reading is OK.

This is very well explained in Steven Shreve: Stochastic Calculus for Finance I, in the first chapters.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.