Value of Call Option as Volatility goes to Infinity

Question

Why would the value of a call option go infinity as volatility goes to infinity?

I understand how you could solve this question by taking $\sigma \rightarrow \infty$ in the solution to the black scholes equation. However, I cannot understand this on a more heuristic level. Surely as volatility goes to infinity you also have a larger chance that the option finishes out of the money (and potentially by a long way)?

Answer 1

The stock price may fall a lot with high volatility, but you can only lose the price of the option if you bought the option. So the upside gets bigger but the downside is bounded.

Answer 2

The value of a call option does not go to infinity as the volatility goes to infinity. It tends to the discounted value of the forward $F=S_0 e^{(r-q)T}$, which when the dividend yield is zero, corresponds to the current value of the stock price $S_0$.

Let me explain why. The value of a call option increases with volatility as the upside to the option is greater if the stock is more volatile - the downside is always floored at zero so this does not change. In the limit of the volatility tending to infinity the value of a call option tends to the stock price. This is already clear from no-arbitrage considerations - you would never pay more than the discounted forward price of the stock price for a call option.

We can see this from the Black-Scholes solution to the call option. This is given by: \begin{equation} C(S_0,T)= S_0 e^{-qT} N(d_1) - K e^{-rT} N(d_2) \end{equation} where \begin{equation} d_1= \frac{1}{\sigma \sqrt{T}} \left[\ln{\left(\frac{S_0}{K}\right)} + \left(r -q + \frac{\sigma^2}{2} \right) T \right] \end{equation} and \begin{equation} d_2= \frac{1}{\sigma \sqrt{T}} \left[\ln{\left(\frac{S_0}{K}\right)} + \left(r - q - \frac{\sigma^2}{2} \right) T \right] \end{equation} When $\sigma \rightarrow \infty$ we have $d_1 \rightarrow \infty$ and $d_2 \rightarrow -\infty$. Hence as $N(x) \rightarrow 1$ and $N(-x) \rightarrow 0$ as $x \rightarrow \infty$, we have \begin{equation} C(S_0,T) \rightarrow S_0 e^{-qT} \end{equation} Understanding why this is the case is not so clear. It relies on us realising that as $\sigma \rightarrow \infty$, the probability distribution for $S_T$ becomes spread out along the whole $S_T>0$ support but with a significant probability mass accumulating on $S_T=0$.

However no-arbitrage conditions require the expectation of $S_T$ at time $T$ must equal the forward price such that \begin{equation} \int_0^{\infty} S_T g(S_T) dS_T = S_0 e^{(r-q)T} \end{equation} where $g(S_T)$ is the probability density function for the terminal stock price $S_T$.

In the Black-Scholes formula the first term is actually the discounted expected value of the in-the-money stock price \begin{equation} e^{-rT} \int_K^{\infty} S_T g(S_T) dS_T = S_0 e^{-qT} - e^{-rT} \int_0^{K} S_T g(S_T) dS_T. \end{equation}

As $\sigma \rightarrow \infty$, the distribution becomes so smeared out that the integral of $S_T$ from $0$ to $K$ is negligible (the only significant probability mass is on $S_T=0$ which does not contribute) compared to the integral from $K$ to $\infty$. Hence the second term on the right hand side becomes negligible by comparison to the first term and so \begin{equation} e^{-rT} \int_K^{\infty} S_T g(S_T) dS_T \rightarrow S_0 e^{-qT} \end{equation} and \begin{equation} \lim_{\sigma \rightarrow \infty} C(S_0,T) \rightarrow S_0 e^{-qT}. \end{equation} We can also show that the put option price converges to the discounted strike. \begin{equation} \lim_{\sigma \rightarrow \infty} P(S_0,T) \rightarrow K e^{-rT}. \end{equation}

Answer 3

Copy pasting parts of an answer I did here as it illustrates the limits of call and put option premia.

$N(d2)$ is the probability that a call option with an exercise price of $K$ is exercised in a risk-neutral world. Therefore, $(1− N(d2)$ or $N(-d2)$ is the probability that a put with the same exercise price will be exercised. Let's plot this as a function of vol.

How about the premium of puts and calls?

Even though there is supposedly zero probability of exercise for a call, I still pays a maximum that seems to be the current spot price, irrespective of the strike. There is another explanation using $N(d1)$ a bit further below in the link I added above (after the PDF of the normal distribution). For the put, however, the maximum is reached at the strike price itself. As @Will explains intuitively, a zero strike call has no vega and does not change when vol is increased to infinity.

At the same time, call option prices are decreasing, as a function of strike (see next chart). Thus, the maximum value a call option can have is that of the zero strike call (which is the price of the current spot when there are no interest rates and dividends). For puts, a similar argument can be made that leads to the strike being the maximum value. I link an answer/explanation where the question is flawed (delta is not the probability of exercise) but the accepted answer is correct.

@Jesper Tidblom's "competition of limits" is also illustrated in the answer I linked.

Answer 4

I also got a bit confused about understanding intuitively what happens in the limit with the Black-Scholes Call and Put values as the volatility goes to infinity, but after analyzing the derivation of the formula it becomes clear.

My confusion was about why the contribution of the $-K$ term disappear in the limit. My faulty reasoning was a bit like this : If $S_T$ in the limit when $\sigma \to \infty$ is supposed to be big most of the time then I felt the option values should be $e^{-rT}(S_0 \cdot e^{rT} - K)$, the discounted payoff of the forward value. And if $S_T$ would be small most of the time, the limit would be $0$. This is wrong.

As LocalVol points out in the comments above, it is important to make a distinction between the size of the underlying and the probability that it has a certain size. The price of the call is (using the risk neutral measure as usual and ignoring the initial discount factor) $$ C = E[Max[(S_T - K, 0)] = E[S_T \cdot 1_{S_T \geq K} ] - E[K \cdot 1_{S_T \geq K}], $$ where we have used indicator functions for the event that we end up in the money. Let us look at the second term first. This is just $$-E[K \cdot 1_{S_T \geq K} ] = -K \cdot \mathbb{P}(S_T \geq K).$$ That is, the value of $-K$ times the risk neutral probablility that this cash flow will occur. 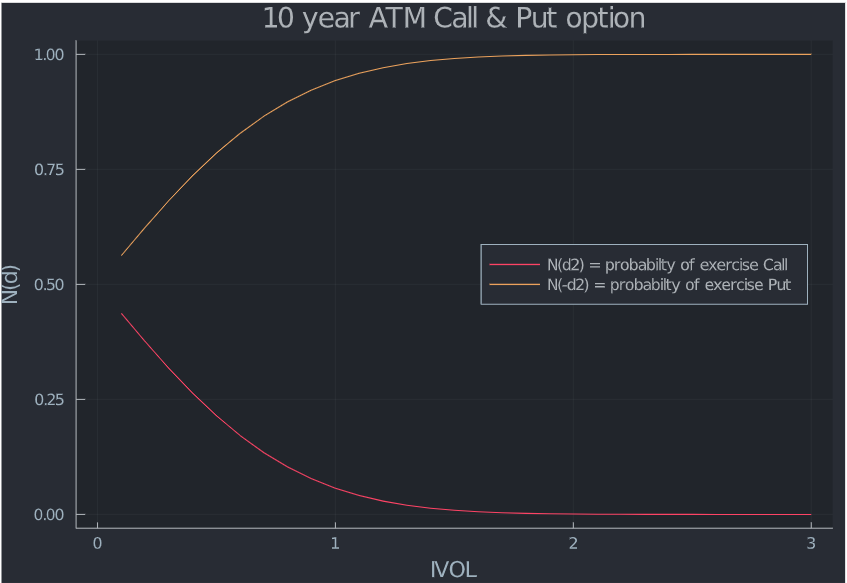I will not rewrite all the math here, but we get that $$ \lim_{\sigma \to \infty} \mathbb{P}(S_T \geq K) = 0. $$ The probablity that we end up in the money goes to zero and the value of this second term goes to zero in the limit. If we were sampling the values of $S_T$, we would find that a larger proportion of paths goes down below K when $\sigma$ grows.

However, the point here is, as LocalVol points out, that although the probability of ending up in the money goes to zero, a larger and larger proportion of the expected value of $S_T$ comes from that region.
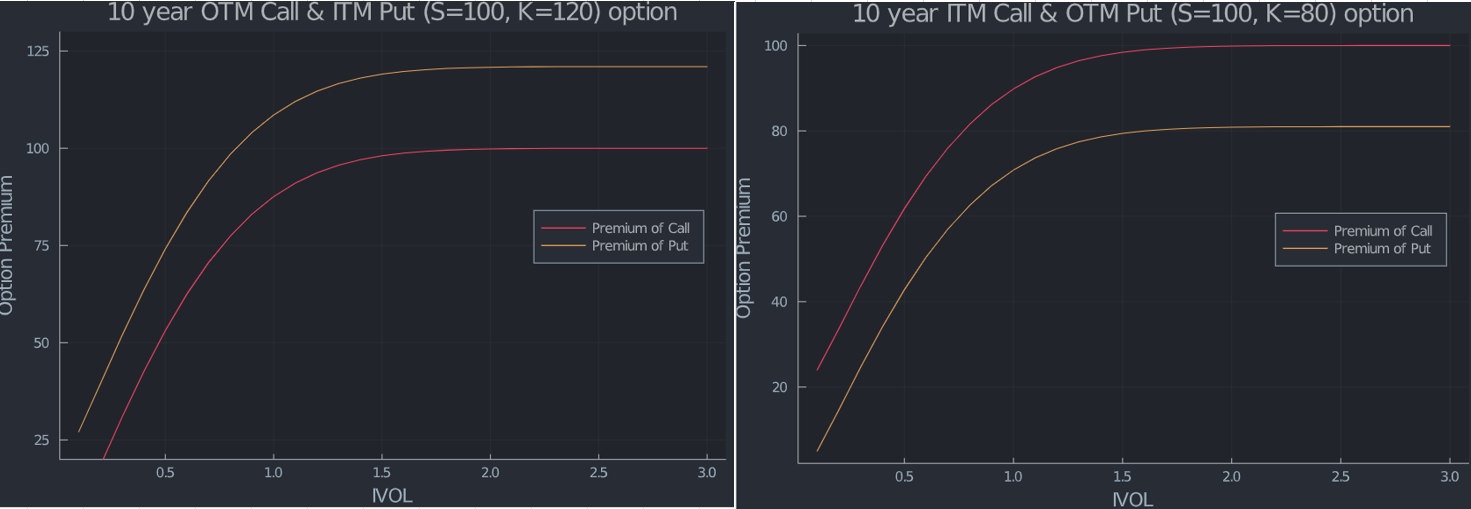
Mathematically we have $$ \begin{eqnarray*} \lim_{\sigma \to \infty} E[S_T \cdot 1_{S_T \geq K} ] &=& S_0 \cdot e^{rT} = E[S_T].\\ \lim_{\sigma \to \infty} E[S_T \cdot 1_{S_T < K} ] &=& 0. \end{eqnarray*} $$ More and more contribution of the expected value come from values of $S_T$ when $S_T \geq K$ as $\sigma$ grows, although the probability for those values to occur goes to zero.

So we have a sort of competition of limits here where the values of $S_T$ above $K$ increases faster than their probability to occur goes to zero, so to speak

Answer 5

When you delta hedge, you make a PnL equivalent to gamma times the difference between implied and realized vol. You want on average the realized vols to average out to the implied vol, in a central limit theorem style:

$Sample mean (Realized vol) = Implied vol$

so you don't make or lose money on average.

Now as vol goes to infinity, the sampling error in the above convergence goes to infinity (it's $vol^2/n$) so you really cannot get convergence. So the only meaningful way now to kill PnL variability is to kill gamma. Hence price is linear in spot (or doesn't depend on spot as in a put option).

Same argument works for when time goes to infinity. The integral of the PnL goes to infinity and thus gamma has to 0.

Answer 6

Just adding another perspective. You have 2 calls at strikes K1 and K2. As vol becomes infinity, the mass between K1 and K2 goes to 0 as the z-scores of K1 and K2 become close to each other. Since there is no mass between them, the K1 call option payoff may as well be flat between K1 and K2.

In other words, the call option at strike K1 is now identical in terms of pricing to strike K2. All such call options have the same price. Which can only be price of the 0 strike option i.e. the discounted forward.