3
$\begingroup$

I refer here to the paper "Betting against beta" by Pedersen and Frazini.

In the model, they construct the following factor, on page 5.

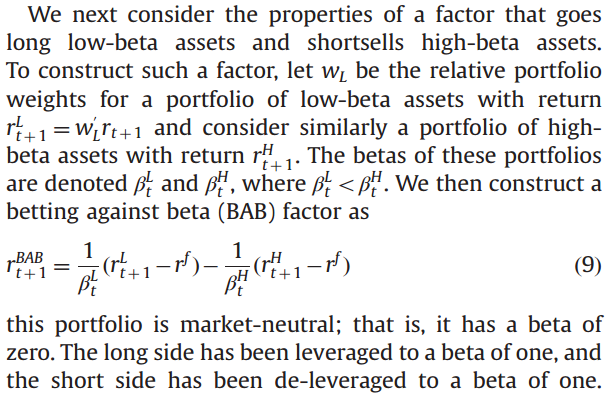
enter image description here

I don't quite understand how this portfolio is being constructed. What do they mean by "leveraging the long side to a beta of 1"? Or deleveraging the short side to a beta of 1?

How exactly does that work in practice? What does leveraging have to do with beta?

As I understand leveraging, we just borrow to finance more investment, right? So what does that have to do with changing beta to become 1?

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ Let's say you have a return such that $R_i - R_f = \alpha_i + \beta_i \left( R_m - R_f \right) + \epsilon_i$. Now imagine you have a return $R_j = 3 R_i - 2 R_f$. Is this a return? If so, what is the market beta for $R_j$? $\endgroup$
    – Matthew Gunn
    Commented Jun 5, 2018 at 17:27
  • $\begingroup$ ........not sure ..................... $\endgroup$
    – Donatello
    Commented Jun 5, 2018 at 17:38
  • $\begingroup$ You would have $\beta_j = 3 \beta_i$. If you $3\times$ leverage something, you're $3\times$ your covariances and $3\times$ your betas (whether they're market betas or something else). $\endgroup$
    – Matthew Gunn
    Commented Jun 5, 2018 at 17:45

1 Answer 1

Reset to default
5
$\begingroup$
  • An excess return is the payoff of a zero cost portfolio. For example:
    • $R_i - R_f$ is an excess return.
    • $c \left( R_i - R_f \right) $ is an excess return for any $c \in \mathbb{R}$,.
    • More generally, $R_i - R_j$ is an excess return for any returns $R_i$ and $R_j$.

Excess returns are nice to work with because you cans simply scale them up or scale them down and they're still excess returns. Let's imagine excess return $R_i - R_f$ has a market beta of $\beta_i$.

$$ R_i - R_f = \alpha_i + \beta_i \left( R_m - R_f \right) + \epsilon_i $$

Then excess return $\frac{1}{\beta_i} (R_i - R_f)$ has a market beta of $1$. $$\frac{1}{\beta_i} \left( R_i - R_f\right) = \frac{\alpha_i}{\beta_i} + \left( R_m - R_f \right) + \frac{\epsilon_i}{\beta_i} $$

Excess return $\frac{1}{\beta_i} (R_i - R_f) -\frac{1}{\beta_j} (R_j - R_f) $ will have a market beta of 0.

Since $\beta_H > 1$, multiplying by $\frac{1}{\beta_H}$ to obtain $\frac{1}{\beta_H} (R_H - R_f)$ is deleveraging the excess return $R_H - R_f$. Since $\beta_L < 1$, multiplying by $\frac{1}{\beta_L}$ to obtain $\frac{1}{\beta_L} (R_L - R_f)$ is leveraging the excess return $R_L - R_f$

Improve this answer
$\endgroup$
2
  • $\begingroup$ So is this correct: Say we have an asset which has beta 0.5. Say we invest two dollars in this asset, and we borrow two dollars to finance it. Then, we have a portfolio which next period will give a return of $2*R_i$ from the asset but take away $2*R_f$ from the borrowing. So our excess return is $2(R_i - R_f)$. But since $R_i - R_f$ was an excess return corresponding to beta 0.5, $2(R_i - R_f)$ has beta corresponding to $1$. $\endgroup$
    – Donatello
    Commented Jun 5, 2018 at 17:47
  • $\begingroup$ @Donatello I think you're on the right track. $\endgroup$
    – Matthew Gunn
    Commented Jun 5, 2018 at 18:11

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.