2
$\begingroup$

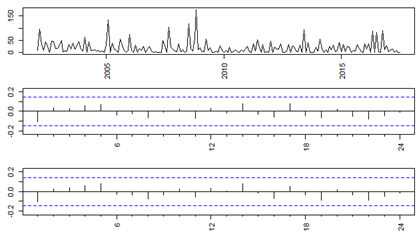
I have an ARIMA model for monthly returns of the brazilian stock market index. Then I test the residuals of the model for ARCH effects. The ACF/PACF of squared residuals show that there are no significant autocorrelations. Portmanteu and McLeod-Li tests also show that there are no heteroscedasticity. Nevertheless, Lagrange Multipler test appear to show there is heteroscedasticity for lags 1 to 8 and the plot of squared residuals itself look a little bit like there might be some volatility clustering. When I fit GARCH models (GARCH, gjrGARCH, AVGarch, TGARCH...), the ones with smallest BIC are (0,1) models, for which the beta is very close to 1 and so the conditional sd forecasts decrease very slowly. This appears to be caused by the fact that no GARCH model should be used, is that correct?

Below: the squared residuals plot, ACF and PACF of squared residuals.

Squared Residuals Plot (first), ACF (second) and PACF (third) of squared residuals

Improve this question
$\endgroup$
2
  • $\begingroup$ can you edit this with the squared residual plot shown $\endgroup$
    – develarist
    Commented Jun 24, 2019 at 8:41
  • $\begingroup$ Thank you for your reply. Edited with the squared residual plot shown. $\endgroup$
    – Paul
    Commented Jun 25, 2019 at 16:42

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.