Are the price of vanilla bull/bear spread constructed by calls and puts same?

Question

We know that both bull and bear can be constructed by either two calls or two puts. Say if given two strikes, will price of bull call equal to price of bull put?

Answer 1

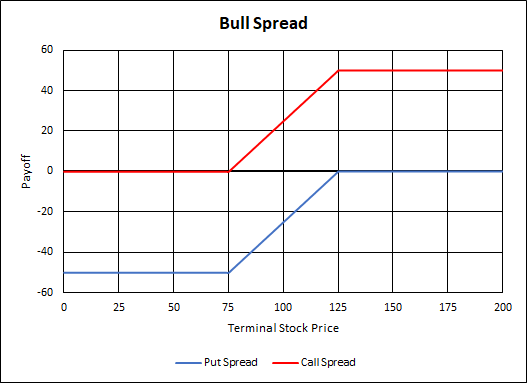
There is a difference between a put spread and a call spread. In the bull case, both strategies expect a mediocre increase in the stock price but the investment idea is different. Look for instance at the two payoffs below for $K_1=75$ and $K_2=125$.

As you see, the call strategy yields a non-negative payoff whereas the put strategy pays at most zero. The difference between both payoffs is $K_2-K_1$. Since this difference is constant for all states of the world, the strategies cannot have the same price (no arbitrage principle): suppose both strategies had the same price and you sold the put strategy and purchased the call strategy. Then, you have zero initial cost but you'll receive $K_2-K_1>0$ at maturity.

The call spread always gives you a positive payoff and hence costs you a positive amount of money when setting the strategy up. I.e. you need to pay a premium (the premium of the lower strike call you buy is higher then the premium of the higher strike call which you sell). It is different for a put payoff which gives you a negative payoff. Hence, you also receive a premium when setting up the strategy.

So, the difference between both strategies is receiving money at the beginning and pocketing it (put) and paying money at the beginning and receiving a payoff at maturity (call).

P.S. Using the put-call-parity, it is of course possible to build an equivalent put spread with call options and then the prices are identical.