Vega of binary option

Question

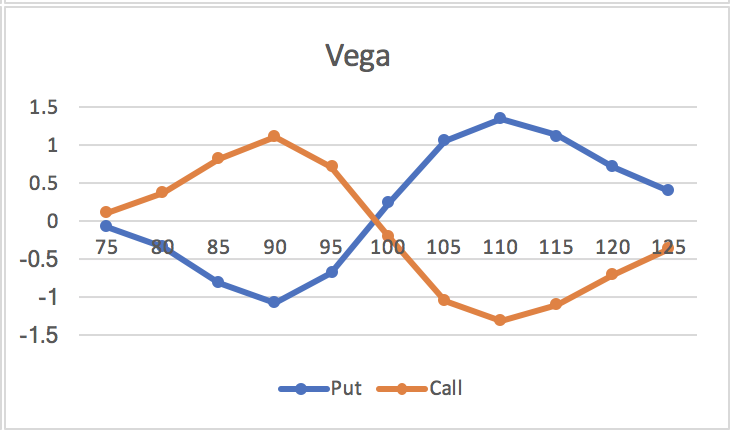
I'm calculating the greeks for a hypothetical binary option, and I'm getting a symmetrical parabola for the vega's of both put and call options that are OTM, ATM, and ITM. Both of them dip into negative territory however. The vega for the call becomes negative when the binary option moves more into-the-money, while the inverse happens for my put.

I read that calls and puts always have positive vegas, which is why I'm confused about my graphed results (see picture; x-axis represents different spot prices, all else equal). Can anyone shed light into this?

Answer 1

A binary call (a similar argument goes for the put) paying $\mathrm{1}_{S_T>K}$ can be seen as the limit of a call spread divided by the difference in strikes as this difference goes to 0:

$\mathrm{1}_{S_T>K} = lim_{dK\rightarrow0}\frac{Max(S_T-(K+\frac{dK}{2}),0)-Max(S_T-(K-\frac{dK}{2}),0)}{dK}$.

Hence it behaves like a call spread. A call spread being a combination of a long option and a short option, it can have a positive or negative Vega, depending mostly (to simplify) on where $S_t$ is relative to $K$.

In fact binaries are typically priced by derivatives traders as call spreads with a certain width depending on various risk factors such as size, liquidity of the underlying etc. In my example the call spread is centered on the strike but obviously this changes in reality depending on whether you're a buyer or seller of the binary (priced as a call spread).