7
$\begingroup$

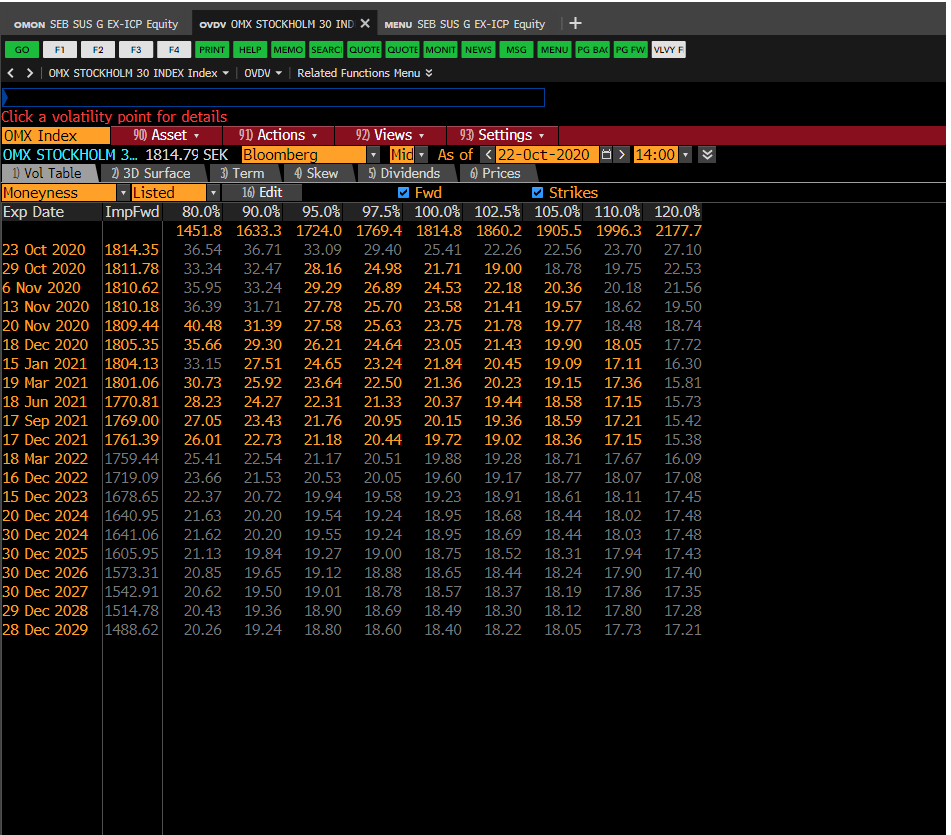
Should be a simple matter, but perhaps I'm misunderstanding something fundamentally. Look first at the below image of the BVOL surface from Bloomberg, to my understanding from looking at the white paper for the surface construction this surface is based on the implied forward of the underlying, which I have taken to mean that the moneyness quoted on the image (95%, 97.5%, 100%, 102.5%...) is the forward moneyness of the option. So what I'm expecting then is that an option with strike = implied forward for a given maturity has the implied volatility of the ATM node, e.g. a Jan 15 2021 option in the picture has an ATM volatility if it's strike is 1804.13 (and not 1814.79 which is the spot price). Playing around in the option valuation tool however I don't see this effect, it then seems that the option that has strike=spot=1814.79 has an implied volatility given by the ATM node at this maturity (that is 21.84%). Am I misunderstanding what it means for a surface to be defined by implied forward moneyness?

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Your are mixing the way the surface is created with what OVDV displays.

OVDV backs out implied forwards and dividends to compute the vol surface as described in the white paper.

However, OVDV itself displays moneyness in terms of spot. It is also easy to verify in your case, where you have Fwd and Strikes ticked. Spot (snapshot at time the surface was built) is 1814.79. All moneyness columns have just one strike. That already suggests it is ATMS. In your case,

  • 100% moneyness is displayed as 1814.8.
  • 90% of 1814.8 is 1633.3,
  • 105% is 1905.5 and so forth.

This is also a natural way for equity, where moneyness is typically referred to as spot moneyness (spot is the underlying after all, and forward are usually unobservable - hence subject to computational assumptions and errors).

There is an exception, when you select to display OVDV in terms of Delta, in which case OVDV uses FWD delta to ensure that 50D call and put will have common strike in this case (and for products priced with Black, where the forward is the underlying). This is again different from OVME which always uses Spot delta.

The help desk (F1F1) should also be able to assist with this question. Ultimately, OVME (and other tools like DLIB) will accurately pull the correct IVOL for the product you are about to price. Since, you cannot download BVOL vols into API without an additional license it doesn’t matter too much what BVOL displays as its not directly useful for pricing. If you feed this into a 3rd party pricer, I would hope Bloomberg enterprise support would help with making sure that you get the desired VOL in the format you require or at least all details that matter for you.

Improve this answer
$\endgroup$
2
  • $\begingroup$ You are saying "OVDV uses FWD delta to ensure that 50D call and put will have common strike". Would not -0,5 Put Delta and +0,5 Call Delta have, by definition, slightly different strikes, because of the discount term P (0, T)? Fwd_Delta_Call = dC/dF = + P(0, T) N(+d1) and Fwd_Delta_Put = dP/dF = - P(0, T) N(-d1) . The only way for d1 (and Strike) to be equal in both cases is for d1=0 and N()=0.5 which makes the deltas different from 0.5. $\endgroup$
    – Vlad Perederiy
    Commented Apr 28 at 14:41
  • 1
    $\begingroup$ No. Put-Call delta parity implies that for forward delta: $\Delta_{call} - \Delta_{put} = 1.0 $, whilst for **spot** delta there is the consideration of discounting: $\Delta_{call} - \Delta_{put} = e^{-r_f t}$. $\endgroup$
    – Attack68 ♦
    Commented Apr 28 at 15:45

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.