Is duration really inversely related to the maturity time length of a bond?

Question

It is always said that longer bonds are more sensitive to interest rates. Intuitively this makes perfect sense, since longer bonds have a larger portion of its cash flow being subjected to stronger interest rate adjustments, and thus their value must be more sensitive to interest rates.

When I tried to prove this quantitatively, things seem to get more complicated. Suppose a bond with par value $a$ pays coupons of amount $y$ annually. If interest rate is $r$, then the value of the bond is.

$$ P=\frac{y}{r}[1-\frac{1}{(1+r)^{n}}]+\frac{a}{(1+r)^{n}} $$

And the relative change in the bond price with respect to interest rate is

$$ \frac{1}{P}\frac{dP}{dr} $$ where $$ \frac{dP}{dr}=y\left\{ [1-(1+r)^{-n}](-r^{-2})-nr^{-1}(1+r)^{-n-1}\right\} -an(1+r)^{-n-1} $$ $$ =\frac{\frac{y}{r^{2}}(1+r-\frac{n}{r})-an}{(1+r)^{n+1}}-\frac{y}{r^{2}} $$ Therefore, $$ \frac{1}{P}\frac{dP}{dr}=\frac{y(1+r-\frac{n}{r}-(1+r)^{n+1})-anr^{2}}{\left\{ yr[(1+r)^{n}-1]+ar^{2}\right\} (1+r)} $$

Suppose long term bonds are really more sensitive to interest rate changes, then the above equation should always be negative, and its value should decrease as n increases. But I am having trouble seem this from the above equation. In fact, numerical results suggest other wise:

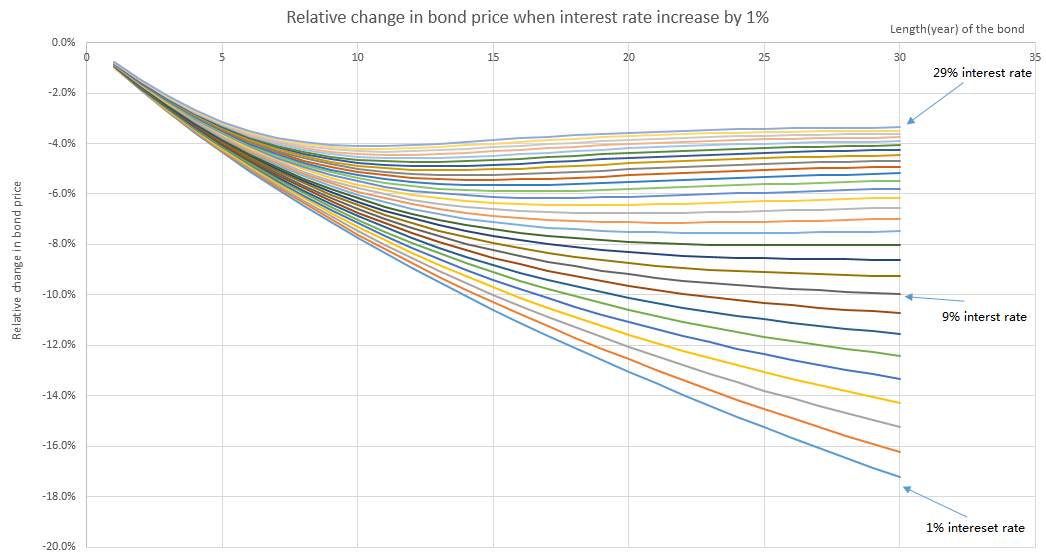
The chart above shows the percentage change in bond price when interest increases by 1%. The bond pays a 6% annual coupon. Each curve represents a bond that is subjected to a different interest rate. For example, the purple curve in the middle represents the relative change in the price of a bond that pays 6% coupon with interest rate being 9%. The vertical axis is the relative change in price, and the horizontal axis is the length of the bond.

We can see from the chart that initially bond price does decrease faster as length increases, but soon reaches a maximum rate of decrease, and eventually the rate seems to move back toward a limiting value.

This leaves 2 questions:

1. With regard to the saying that "longer bonds are more sensitive to interest rates", is this a statement that is true for most practical cases, or is it a statement that's always true mathematically?

2. How can we algebraically derive the results seen in the chart to answer questions such as where do the maximum rate of decrease occur, and what is the limiting value, if it exists at all?

Answer 1

Assume a number of bonds with three constant variables, par value $par$, coupon value $C$ (paid annually), and interest rate $r$, and one changing variable, time to maturity $n$

First off, the relevant formulas:

The price $P$ of each bond, as you've already written it, is

$$ P=C*[\frac{1}{r}-\frac{1}{r}*\frac{1}{(1+r)^{n}}]+\frac{par}{(1+r)^{n}} $$

The duration $D$ of each bond is

$$ D = \sum_{t=1}^{n}{w_t*t} $$

where $w_t$ is

$$ w_t = \frac{C_t}{(1+r)^t}*\frac{1}{P} $$

The volatility $V$ (modified duration) of each bond is

$$ V = \frac{D}{(1+r)} $$

The modified duration $V$ gives an exact measure of the bond's exposure to interest rates. Thus the bond price sensitivity to interest rates is

$$ \frac{\Delta P}{P} = -V*\Delta r $$

Now if you put all these together it should easily follow that "the longer the bond the higher the sensitivity to interest rates".

First off, it's probably easy to see how $P$ relates to $n$. As $n$ goes up $P$ goes up as well. As $n$ gets bigger however the price increase rate drops and you eventually get closer and closer to $\frac{C}{r}$ (perpetuity formula). You do however constantly move up.

Similarly, as $n$ gets bigger, the bond duration $D$ increases as well. It also approaches a limit but it continuously increases.

By now you should probably see where I'm going with this. As $D$ gets bigger, $V$ gets bigger as well. As $V$ gets bigger the bond price sensitivity to interest rates goes up as well.

It follows then that as $n$ gets bigger (the bond becomes 'longer') the bond price sensitivity to rates goes up as well.

To see a numerical example as well have a look at the following charts:

$C = 100,\ par = 1000,\ r = 0.05,\ \Delta r = 0.001$

$P(n)$

$D(n)$

$\frac{\Delta P}{P}$ for $0.001$ increase in $r$ (the chart says "0.01 dr". That is wrong. The change in r is 0.001)

$\frac{\Delta P}{P}$ for $0.01$ increase in $r$ for multiple $r$ levels

Ultimately I end up with a graph similar to yours but with no surprising results. There's no bond price change line curving up. All lines get closer and closer to their limit and then move to the right almost horizontally.

To wrap it up in terms of algebraic computations and thus address your second question as well we have the following:

$$ \lim_{n \to +\infty}P = \frac{C}{r} $$

$$ \lim_{n \to +\infty}D = 1+\frac{1}{r} $$

$$ \lim_{n \to +\infty}V = \frac{1}{r} $$

Mathematically speaking, the formulas confirm the answer to the first question for all numbers, not just practical ones, and they also allow you to calculate the relevant limits.

The numbers and charts used in the numerical example above conform to these limit formulas as well. The bond price approaches $2000$ which as per the above formula is $100/0.05$, the bond duration approaches $21$ which is $1 + 1/0.05$, volatility approaches $20$ which is $1/0.05$, and the bond price sensitivity to interest rates approaches $-0.02$ which is $0.001*(-20)$

Answer 2

I'm not a bond trader and haven't looked at this in years, so my quantities may not be defined exactly as per convention, but it is generally correct.

To answer your question, you should restate the present value of the bond using exponentials. This new formulation is exactly equivalent to what you wrote but much more tractable algebraically (note that my interest rate $r$ below is not exactly the same as yours, it needs to be adjusted using the usual methods).

So:

$$ P = y \Sigma e^{-rt_n} + a e^{-rt_N} $$

Now this is trivial to differentiate:

$$ \frac{1}{P}\frac{dP}{dr} = \frac{y \Sigma t_n e^{-rt_n} + a t_N e^{-rt_N} }{P} = D ! $$

Bingo, that's the definition of your bond's duration right there!

The derivative of your bond's PV is the weighted payout time of its coupons. This is an exact relationship, and not just an approximation.