These are two simple concepts that address a practical problem when settling coupons that are only calculated in-arrears. Let us assume that $r_i$ is the SOFR fixing valid for business day $i$ (up until next bus day, $i+1$). Denote by $n_i$ the number of calendar days between $i$ and $i+1$, divided by the appropriate nbr of days in a year (e.g. 360 or 365).
Assume that you wish to calculate the coupon for the accrual period from $t$ to $T$, that is also due to be paid at time $T$. Now you seek to compound the daily SOFR rates in order to determine the payment amount; but you will realize that the last fixing (the one from $T-1$, as the accrual end date is excluded from compounding) will be available to you "too late" (usually at $T$, depending on which time zone you sit in & because central banks usually publish these rates with a delay). This is too little time for counterparties to reconcile numbers and settle payments at $T$. Hence, you have two (or more) options, to work around this problem:
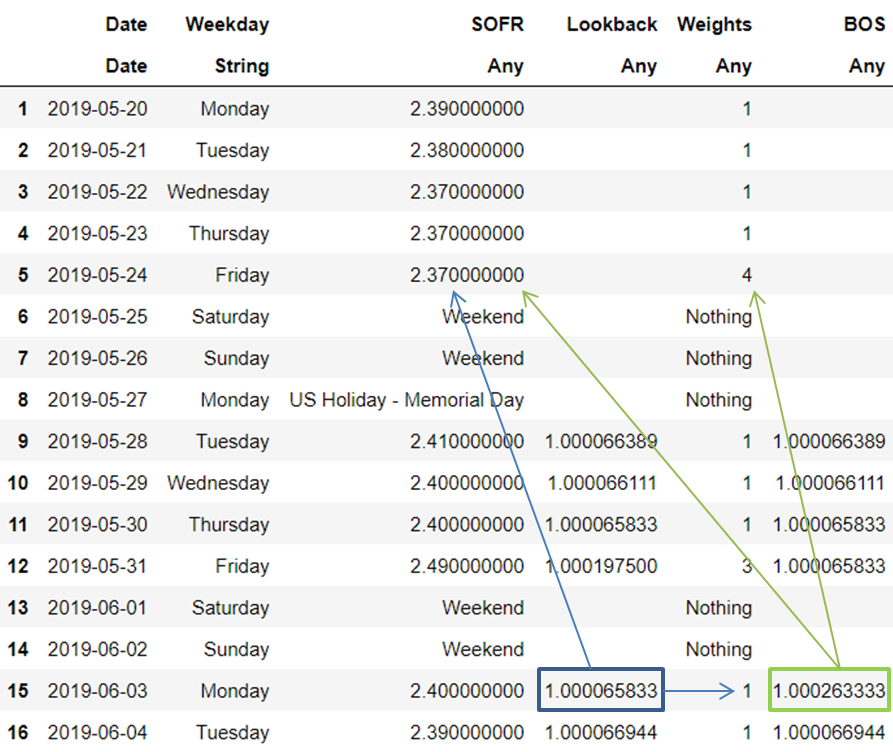
a $k$-day lookback (without observation shift): $$ \Pi_{i=t}^{T-1} (1 + r_{i-k} n_i) -1 $$ In simple words, for compounding the rate of Wednesday, you simply use the SOFR fixing that happened $k$ days before this Wednesday. Do note that there is also a variant of this, where you also shift the day-count weights of the fixing, leading to $ \Pi_{i=t}^{T-1} (1 + r_{i-k} n_{i-k}) -1 $. In both ways, you know the final rate now $k$ days ahead of payment.
a $k$-day lockout: $$ \Pi_{i=t}^{T-1-k-1} (1 + r_i n_i) \Pi_{i=T-1-k}^{T-1} (1 + r_{i-k} n_i) -1 $$ This simply means that you take the rate from $k$ days before the accrual end date and use this constant value for the remainder of the period. Also in this case, you know the final rate now $k$ days ahead of payment.
Hope this helps.