I run a MVP on 10 ETFs: SPY, SDY, IWB, XLP, VGT, BND, XLF, IJR, XLY, XLI from 2008 to 2016 on monthly return data. The weighs array (I am using a MATLAB function "Portfolio" - constraints are simple: no shorts + weights sum to 1) gives me only 1-3 of the 10 ETFs only - the rest are zero weight.
Does this seem intuitive?
Thanks,
Andrew
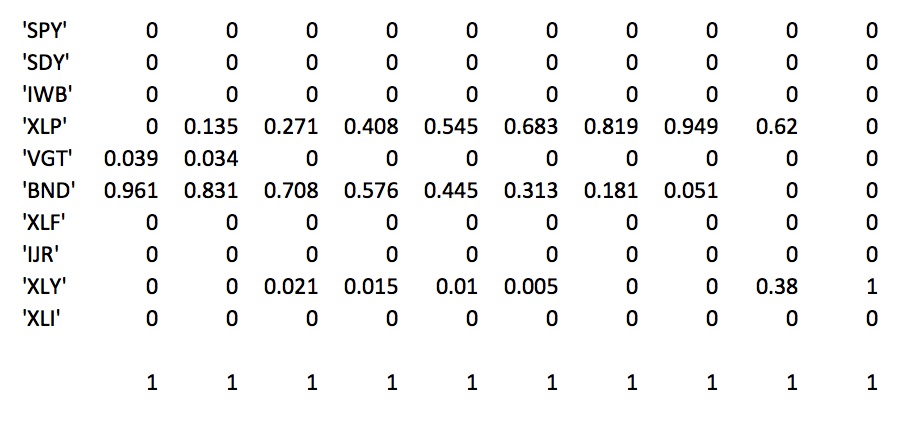
The long only minimum variance portfolio is either equal to the unconstrained minimum variance portfolio, which is usually dense, or it is guaranteed to be sparse - in the sense that there are only few nonzero coefficients. This is a consequence of the fact that the minimum is either achieved at the global minimum or at one of the corners of a convex polyhedron. A typical example for a dense long only portfolio result is from a diagonal covariance matrix.
At first this looks counterintiutive, as one intuitively equates low variance with low risk, and low risk and a sparse portfolio seems like a contradiction. But this method only looks at volatility - other aspects like default risk or so are ignored.
The usual trick is to increase the density of the resulting portfolio is to add a constant to the diagonal of the covariance matrix, thus making it more diagonal. The effect is to make the resulting portfolio more similar to the equal weight portfolio and thus less sparse.
The output you have given looks more like an 'efficient frontier' than a minimum-variance portfolio, but in general, long-only minimum-variance portfolios tend to be concentrated in only a few assets, in particular when i) the marginal volatilities in the asset universe are very different (then, the low-vol assets will be included) and ii) when correlation between the assets is high.
