Market impact, why square root?

Question

The standard method of market impact is the square-root formula \begin{equation} \Delta P = c \cdot\sigma \cdot \sqrt{\frac{n}{\nu}} \end{equation} where $\Delta P$ is the price change from executing a trade for $n$ shares, with market volatility $\sigma$, average market turnover $\nu$ and some constant $c$. This is empirically justified across a wide range of markets (even Bitcoin).

Is there any good theoretical justification as to why the square-root formula is so consistent across different markets?

Answer 1

I found this power point and this paper to be an excellent source on this topic.

Here is a quote from the paper:

A square-root singularity for small traded volumes is highly non-trivial, and certainly not accounted for in Kyle’s classical model of impact [11], which predicts a linear impact ∆ ∝ Q. A concave impact function is often thought of as a saturation of impact for large volumes. We believe that the emphasis should rather be placed on the anomalous high impact of small trades. Numerically, Eq. (1) means that trading one hundredth of the daily volume moves the price by a tenth of its daily volatility, which is indeed a huge amplification. Mathematically, Eq. (1) implies that marginal impact diverges for small volumes as $Q^{-1/2}$ , meaning that the susceptibility of the market to trades of vanishing size is formally infinite. In most systems, the response to a small perturbation is linear, i.e. small disturbances lead to small effects. The breakdown of linear response often implies that the system is at, or close to, a critical point, where very special properties emerge, such as long-range memory or scale invariant avalanches, that accompany this diverging susceptibility.

It goes on to say that besides being empirically robust (it appears to hold in a suprisingly wide number of settings), the square root law arises according to the authors from the very peculiar nature of the order book (the collection of all buy and sell orders) near the boundary between buying and selling. Closer to the "current price", the order book rapidly thins in density.

Indeed if this thinning in price-space of the order book is approximately linear, then the window in price space required to fill an order of dollar size Q will grow with the square root of Q (my own illustration):

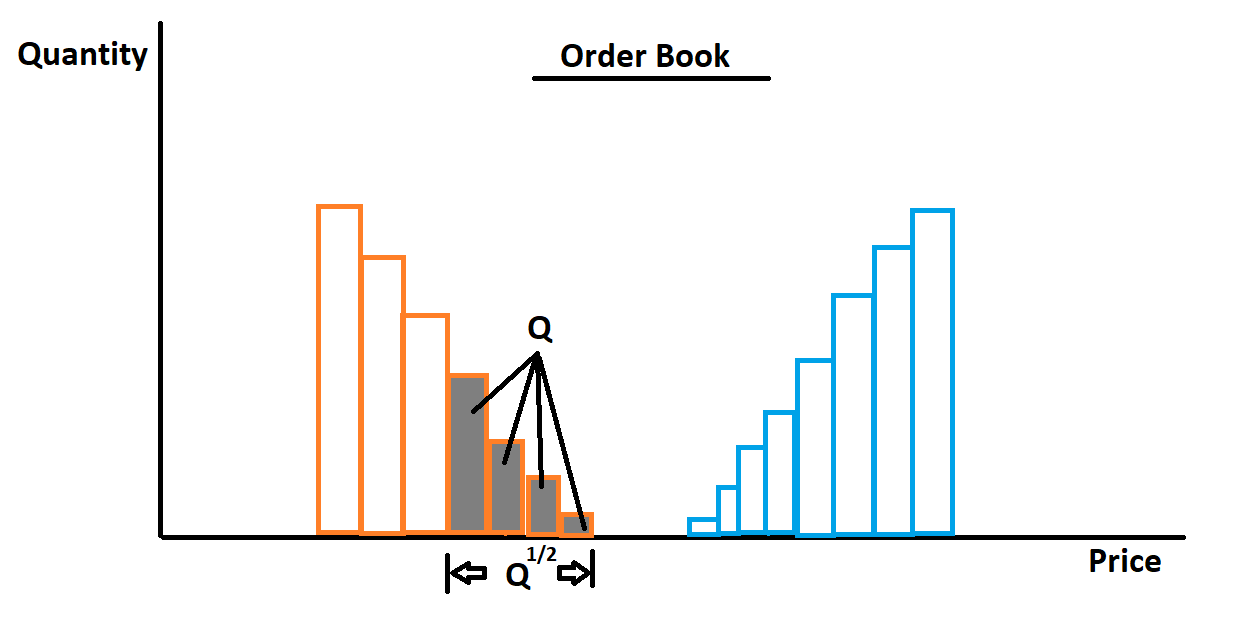
Their model to explain this thinning supposes that orders undergo a diffusion process in price space (a diffusion associated with volatility), and therefore, the order book thins in density near the critical point where buy and sell orders meet each other and annihilate (execute).

Answer 2

My understanding (devoid of any mathematical grounding) is as follows.

v = Turnover PER UNIT TIME
n = Shares you need to execute

therefore

n/v = Number of units of time required to execute your size at the normal turnover rate

---

Realized vol follows a SQRT(T) heuristic.

Given that we can now rewrite the transaction cost formula purely in terms of vol and time units.

This translates to the observation that the cost of execution over a period is directly proportional to the realized vol over the period