Intuition for the Effect of Vol of Vol in Heston Model on Volatility Surface

Question

I was hoping someone could describe the economic/mathematical intuition behind the effect that the vol of vol parameter has on the volatility surface, in particular the slope to maturity. Take for instance, as in Kienitz and Wetterau (2012), the model as $$dS(t)=\mu S(t)dt+\sqrt{V(t)}S(t)dW_1(t)$$ $$dV(t)=\kappa(\Theta-V(t))dt+\nu\sqrt{V(t)}dW_2(t)$$ $$S(0)=S_0$$ $$V(0)=V_0$$ $$\langle\,dW_1,dW_2\rangle=\rho dt$$

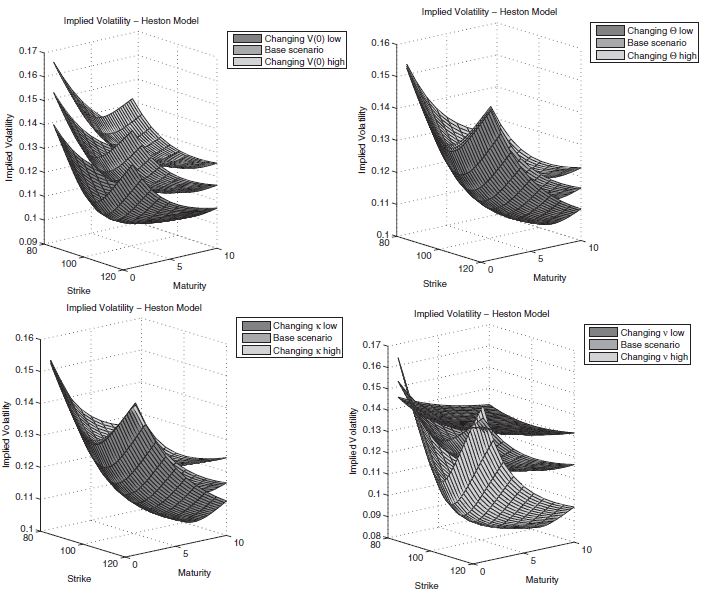
The authors then supply the following vol surfaces after perturbing certain parameters:

All of these make sense to me accept the final chart showing the effects of perturbing the vol of vol, $\nu$. The more pronounced smiles at any given maturity are fine, I get those. I may be missing something obvious, but how does a higher vol of vol, all else equal, lead to declining option prices as we extend the maturity?

Answer 1

Maybe it would help you to think of it the following way.

The strike $\sigma^2(T)$ of a fresh-start variance swap of maturity $T$ in the Heston model only depends on parameters $(v_0,\theta,\kappa)$, see related question here. More specifically

\begin{align} \sigma^2(T) &= \Bbb{E}_0^\Bbb{Q}\left[ \frac{1}{T} \langle \ln S\rangle_T \right] \\ &= \theta + (v_0-\theta) \frac{1-e^{-\kappa T}}{\kappa T} \end{align}

A well-known model-free result is that one can express the above variance strike as an integral over strike space of weighted OTMF option prices (see here), or equivalently, as an integral over strike space of the implied volatility smile (actually a slight re-parameterisation of it, see here)

Now, you seem to be OK with the fact that when you increase (resp. decrease) vol of vol in Heston, the convexity of the IV smile at any given maturity is expected to increases (resp. decreases).

From all the information above, we can then add that, for any given smile of maturity $T$

• When you decrease vol of vol, the convexity of the smile decreases. Because $\sigma^2(T)$ needs to stay the same however (you did not change $v_0$, $\theta$ or $\kappa$), the ATM volatility level then mechanically needs to increase so that the integral of vol in strike space remains the same.
• When you increase vol of vol, the convexity of the smile increases. Because $\sigma^2(T)$ needs to stay the same however, the ATM volatility level then mechanically needs to decrease so that the integral of vol in strike space remains the same.