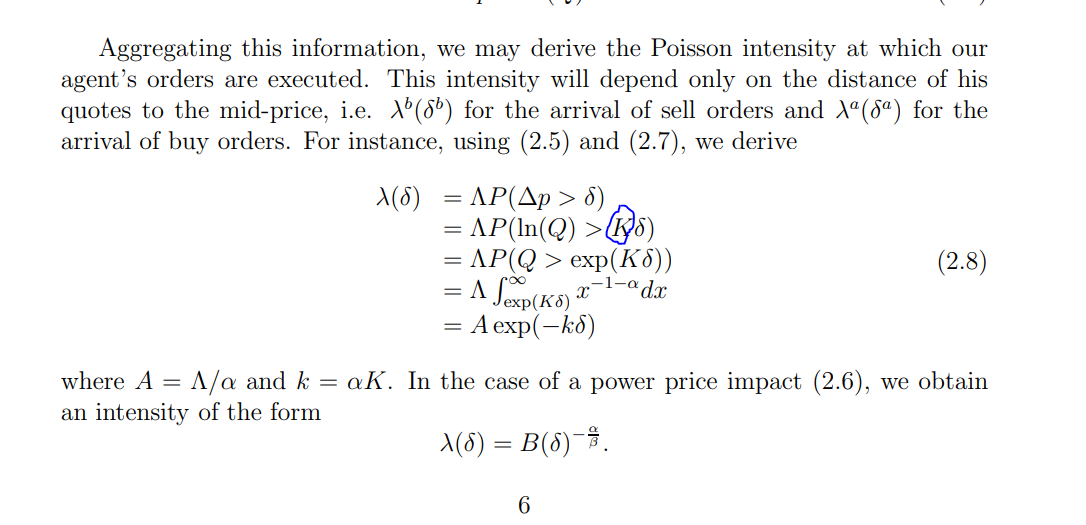
Where does the K term come from in Avellaneda's description of finding the probability an order gets filled. Please see the image below
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
It is a consequence of (direct) proportionality from equation (2.10):
Equation (2.10) states that the change in price ($\Delta p)$ is proportional to the logarithm of the market order size $Q$:
$$ \Delta p \propto \ln(Q). $$
From the definition of direct proportionality, $\Delta p$ is directly proportional to $\ln(Q)$ if there exists a non-zero constant $c$ such that:
$$ \Delta p = c \cdot\ln(Q) $$ where $c$ is known as the proportionality constant. Replacing $\Delta p$ with $c \cdot\ln(Q)$, dividing with $1/c$ on both sides and setting $1/c = K$, yields the result of the second equation in the derivation:
\begin{align*} \mathbb{P}\left(\Delta p > \delta\right) &= \mathbb{P}\left(\ln(Q) \cdot c > \delta\right)\\ &=\mathbb{P}\left(\ln(Q) > \frac{1}{c} \delta\right)\\ &=\mathbb{P}\left(\ln(Q) > K \delta\right). \end{align*}
A discussion regarding estimation of the model can be found here.
-
$\begingroup$ Basically I need to fit 1/c to make deltaP proportional to c*ln(Q), based on historical data? $\endgroup$ Commented Dec 14, 2022 at 18:52
-
$\begingroup$ @OscarMorales You would need to fit the end result of the equation ie. $\lambda(\delta)=A \exp(-k \delta)$, with $k$ and $A$ be defined as above. You then try to estimate the params in this equation, $\alpha$, $K$, $\Lambda$ (AFAIK). I answer the question of where $K$ originates from, which might be from the definition of proportionality. Remember that 1/c is still a constant (for $c$ being a constant) and can be redefined as $K$ to fit the premise of the above equation. I've only read the paper and never tried to fit the model. Therefore, I don't have much insight into this premise. $\endgroup$– PlebCommented Dec 14, 2022 at 19:28
-
$\begingroup$ Got it this makes sense, thank you. $\endgroup$ Commented Dec 14, 2022 at 20:50