I am trying to understand the intuitive reasoning for why volatility is more for deep OTM/ITM put/call then ATM..(why Simles for equity) Why ATM will not have more volatility as deep OTM/ITM option will be less likely to be exercised..
Thanks for the help!!
There are several reasons, maybe the most important and also quite intuitive one: Implied volatility more or less assumes that the stock price is driven by Brownian motion and thus moves in a continuous fashion.
What we observe is that stocks can jump (usually downwards, sometimes upwards) which needs to be modelled using something like a jump process (maybe in addition to some diffusion part).
This possibility of jumps leads to prices of OTM options to be relatively high. In order to account for this high price we need a relatively high implied vol.
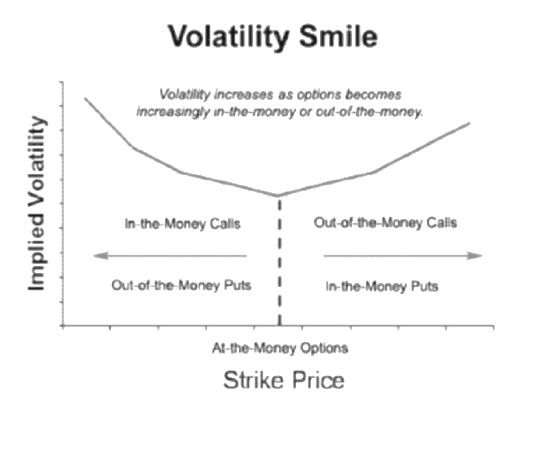
Too many people think implied vol is the "volatility" that you think it is. Normally we talk about "volatility" as the measure of how unpredictable the stock movement will be in its magnitude. True.
Now, detach "implied vol" from the definition of "volatility". Now, just imagine that "implied vol" is just a parameter of B-S model. What trades in the market are option prices. There is a relationship between these prices and "implied vol" and this relationship is important when you use B-S model. When you are fixated on the B-S model and you believe this is your bible, then the market prices dictate that there is a smile. This is the reason for the smile.
But seriously what is implied vol? it's just a concept, you can call it anything whatever. It is not the same as real volatility.
As Richard mentioned, in some particular Markets for a given expiration, options whose strike price differs substantially from the underlying asset's price command higher prices than what is suggested by standard option pricing models. These options are said to be either deep in-the-money or out-the-money. Graphing implied volatilities against strike prices for a given expiry yields a skewed "smile" instead of the expected flat surface.f you plot the implied volatilities against the strike prices, you might get the following U-shaped curve resembling a smile. Hence, this particular volatility skew pattern is better known as the volatility smile.
In fact,Volatility smiles tell us that demand is greater for options that are in-the-money or out-of-the-money.
The volatility smile is the result of market forces knowing form experience that out of the money option pay out more often that what would be expected by a normal (Gaussian) distribution. For years Quants speculated why the market drove the out of the money options higher that the price of the Black-Scholes model.
The best theory speculates that the smile is because the distribution of returns of stock prices are not a normal distribution having large jumps in price that occur too often. The distribution is much closer to a power law distribution or Pareto distribution.
The problem is that the math becomes messy without the normal distribution so the most recent models that include smile uses a jump diffusion process ( a normal distribution with random jumps).
