Proof of approximation formulas for implied volatilities

Question

I am trying to calibrate a local volatility model to observed implied volatility smiles (not surfaces!, just a smile given for fixed maturity).

I ran into the following approximation, and thought I could plug in my implied volatility formula, and solve for local volatility; $$\sigma_{i}^2(K,T) = \frac{\sigma^2(S(0), 0) + \sigma^2(K,T)}{2}.$$

$\sigma_i$ is implied volatility.

My question however is, where does this approximation come from?

I know Dupire's formula, you know the one that is a fraction of two integrals. I can't quite find it, but is it from that the approx is derived? If so, how?

Answer 1

Assume the local volatility dynamics is: $$dS_t/S_t = \sigma(S_t) dW_t^\Bbb{Q} $$ where we have just assumed $r=0$ without loss of generality (if $r$ is not zero, you just need a change of measure to the $T$-forward measure).

Suppose you would like to fit the market implied volatility smile $\Sigma(T,K)$.

There is no closed-form formula to express $\Sigma(T,K)$ as a function of $\sigma(S_t)$.

There are however a bunch of approximations available in the literature:

• For short term options ($T \to 0$), we have $$ \Sigma(T,K) \approxeq \frac{\ln(S_0/K)}{\int_K^{S_0} (s\sigma(s))^{-1} ds} $$ known as the BFF approximation (Berestycki, Busca & Florent - 2001). You can obtain this formulation by (1) expanding Dupire local volatility in time (2) assuming that the implied volatility can also be expanded in powers of time and identifying zero-th order in $T$ terms.

• For longer term options, one can expand the latter formula to obtain: $$ \Sigma(T,K) \approxeq \Sigma_0(K) + \Sigma_1(K) T $$ with $$ \Sigma_0(K) = \frac{\ln(S_0/K)}{\int_K^{S_0} (s \sigma(s))^{-1} ds} $$ $$ \Sigma_1(K) = -\frac{\Sigma_0(K)}{\left( \int_K^{S_0} (s\sigma(s))^{-1} ds \right)^2} \ln \left( \frac{\Sigma_0(K)}{\sqrt{\sigma(S_0)\sigma(K)}} \right) $$

• For short term options another approximation is due to Pat Hagan (Hagan, Kumar, Lesniewski & Woodward - 2002) it reads: $$ \Sigma(T,K) \approxeq \sigma(\sqrt{S_0 K} ) $$

References

1. Berestycki, Busca, Florent. (2001). Asymptotics and calibration of local volatility models. Electronic copy available here.

2. Hagan, Kumar, Lesniewski, Woodward. (2002). Managing smile risk. Wilmott Magazine, 84-108. Electronic copy available here

Answer 2

@Quantuple's answer is correct. There is no exact closed-form formula for call prices or implied vols as a function of local vols, which is unique and ironic, given there are formulas the other way around. The closest thing to a practical exact formula, to my knowledge, is the harmonic short maturity expansion given in the answer.

However, (and this is a direct answer to your question), the so-called "sigma-zero" formula does provide an exact expression for the implied vol as a function of the local vols, although this is an implicit formula, and the evaluation of some of its terms requires a numerical implementation. Hence, this formula, despite being exact, is not directly usable in practice.

Here is the formula (this is a more general formula that also works with stochastic volatility, for local volatility, replace the conditional expectation of the local variance by the square of the local vol):

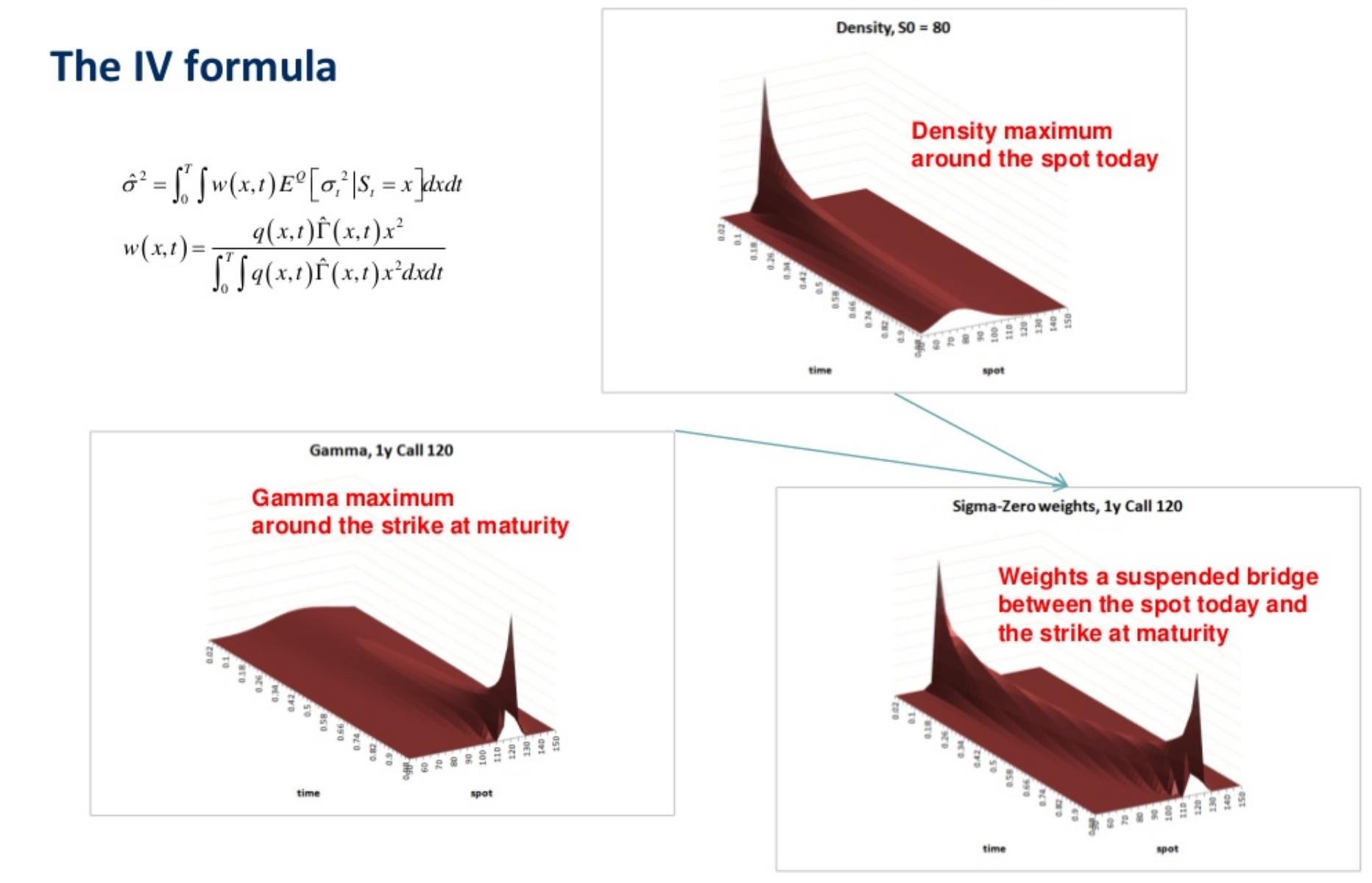
• The implied variance is a weighted average of local variances over time and spots.
• The weights are the product of the probability density (which is not known analytically in local vol models and must be computed numerically, for instance with FDM over the Fokker-Planck equation) by the gamma (computed in Black-Scholes with the resulting implied vol, making the formula implicit).

For a demonstration, please see this video: Antoine Savine, RiO2018 or access its slides on SlideShare. You may also consult my lecture notes here, slides 71-78.

Note that the sigma-zero formula was also found (but not published) by Dupire, and his demonstration is particularly enlightening, in what it comes from an analysis of hedge errors.

Despite its impracticality, this formula offers a deep intuition of how local vols combine to produce European option prices and implied vols, and it has been the basis of a vast amount of work to find more or less precise, more or less complicated practical approximations, most prominently by Blacher (see his talk on RiO 2018 on YouTube) and Gatheral (in his famous textbook Volatility Surface).

By far the simplest (but definitely not the most accurate!!) of these approximations is to note, as seen on the picture, that probability density is maximal on the spot today, whereas gamma is maximum at the strike at maturity, hence a simple approximation is to average local variances from these two points, ignoring the rest of the surface.

And this exactly is the approximation you are asking about :)