pure factor return for factor model

Question

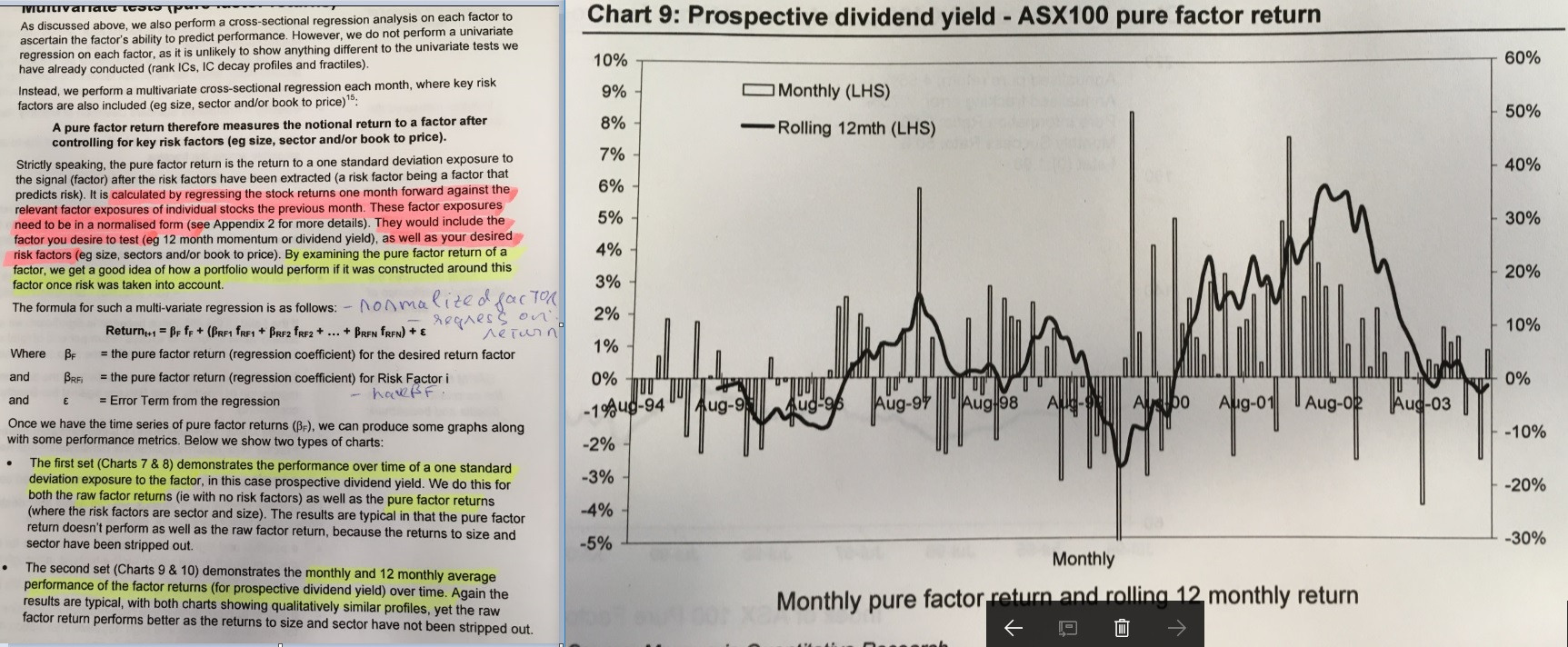
I am reading a paper. The authors use the multivariate regression to calculate the pure factor return $\beta_F$ using the following equation: $$Return_{t+1}=\beta_F f_F + \beta_{RF_1} f_{RF_1} +⋯+ \beta_{RF_N} f_{RF_N} +\epsilon$$

where

• $\beta_F$ = pure factor return for the desired return factor,

• $\beta_{RF_i}$ = pure factor return for Risk factor $i$,

• $f_F$ = evaluated factors (ex: Dividend Yield)

• $f_{RF_i}$ = risk factor (ex: size)

After that, they produce a monthly factor return as the graph here. I just wonder from the pure factor return for the desired return factor, how they can get the return over months like the graph?

Answer 1

From that picture you took, it looks like the $\beta_F$ is a time series. It's computed by doing a cross-sectional regression at each point in time.

Answer 2

In the cross sectional framework, the "pure factor return" is actually the return of the characteristic portfolio, which has unity exposure to the underlying factor and zero to others. If you hold the portfolio for one month, you get the monthly return.