0
$\begingroup$

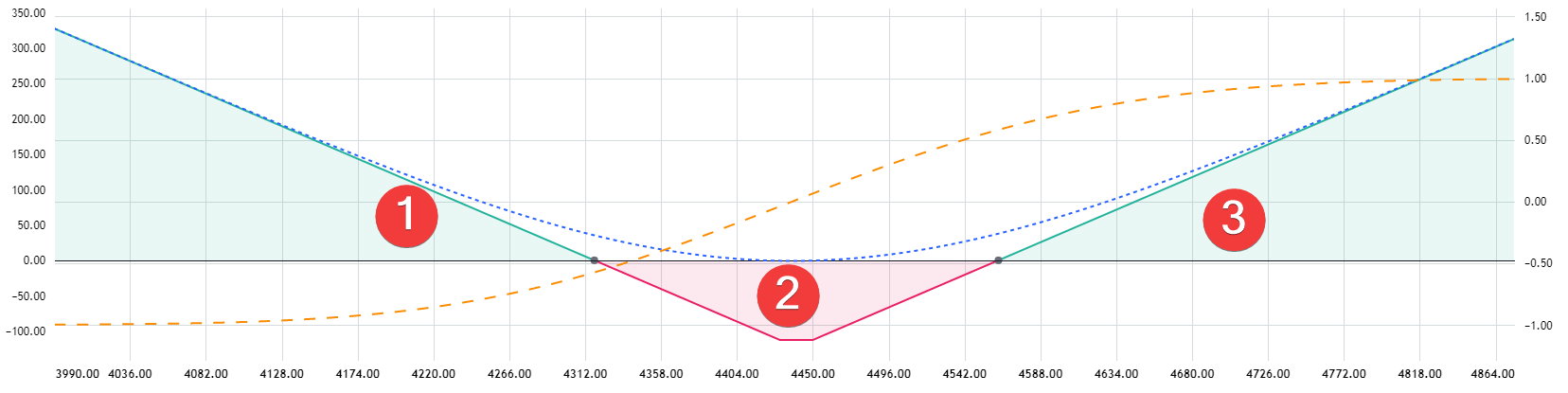
Big picture

For any options strategy, for any segment between zero profit (breakeven) points, I want to calculate probabilities of the underlying instrument price will be within a segment at expiration. On the illustration, I want to know probabilities for segments 1,2 and 3.

enter image description here

My environment

  • No time series of the underlying instrument price available. So I don't consider approaches where I have to fit real distribution with something like Student.
  • Analitical form of a volatility curve is available.

The approach that is on my mind

  • For each breakeven point calculate a delta of a virtual option with a strike at this point. Can do this since I have analitycal IV curve.
  • Each of these deltas can be interpreted as a probability of underlying price is higher then corresponding breakeven point at expiration
  • Having array of these probabilities, I can calculate probabilities for segments.

My questions

  • Is my approach generaly acceptable?
  • What are another approaches you might advise?
Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ For this interpretation to be useful, each assumption must remain static from the start of the trade until expiration. That is not realistic. For a snapshot, it could be useful depending on the rest of the assumptions that went into your analytical model. Either way, this is probably too full of assumptions to be relied upon, IMO. $\endgroup$
    – amdopt
    Commented Jun 21, 2023 at 11:13
  • 5
    $\begingroup$ Hi, if you have the volatility available, you can calculate the implied probability density and integrate that. quant.stackexchange.com/questions/29524/… $\endgroup$
    – Kermittfrog
    Commented Jun 21, 2023 at 11:15
  • 1
    $\begingroup$ I second @Kermittfrogs comment and would like to add that delta is only a rough proxy. $\endgroup$
    – AKdemy
    Commented Jun 21, 2023 at 12:03
  • $\begingroup$ @kermittfrog following your link I found this. Seems reasonable and not hard to implement in my case. The only thing that is not clear for me now, is how to integrate the most left segment (from -inf). Thank you all for the comments, it helped me. $\endgroup$
    – plkn
    Commented Jun 21, 2023 at 13:42
  • 2
    $\begingroup$ As a warming: you are looking at risk neutral (implied) probabilities. The information content with regards to real world probabilities can be very low. In other words, I would be very careful about trying to make investment decision based on these "probabilities". $\endgroup$
    – AKdemy
    Commented Jun 21, 2023 at 16:06

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.