-3
$\begingroup$

I have the solution as given 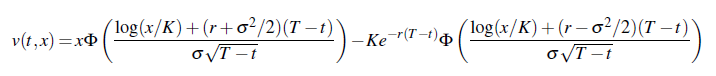enter image description here

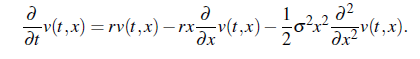
Based on this, I have to show that this solves the Black-Scholes formula enter image description here

It means that I should take the partial derivatives of the solution above and then receive the differential equation of Black-Scholes.

Anyone can give me an intuition how should I do that? Should I use Ito's lemma to compute the derivatives?

Improve this question
$\endgroup$
1
  • $\begingroup$ Maybe just ask Mr. Kallsen or your tutor. $\endgroup$
    – user16299
    Commented May 20, 2015 at 17:22

1 Answer 1

Reset to default
4
$\begingroup$

The above equation is the price of a call option. It has nothing stochastic inside it. It only depends on the current price and the time. So no Ito is needed. You should just compute the derivatives of your solution v (like you do for any deterministic multivariable function), plug them into the PDE and verify that it's satisfied.

Improve this answer
$\endgroup$
4
  • $\begingroup$ may be you could show me how to differentiate the 1st term with respect to t. thanks $\endgroup$
    – 054
    Commented May 18, 2015 at 20:12
  • $\begingroup$ @054 Showing it by typing is tedious. I'm 100% sure I've seen it in standard text-books. Actually you'll get it by simple google. $\endgroup$
    – SmallChess
    Commented May 19, 2015 at 0:10
  • $\begingroup$ Before downvoting try to help. There is nothing similar in the Internet. This case is special as it has a product of variable and the cdf of normal distribution again oft the same variable. The stack exchange sites are part oft the Internet too. $\endgroup$
    – 054
    Commented May 19, 2015 at 15:09
  • 4
    $\begingroup$ You're getting downvoted because after receiving good help you just ask for more instead of showing any work yourself. Your question looks like homework and our users are generally encouraged to solve that themselves as much as possible. For the sake of the people that answer but also for their own: you learn most by doing. $\endgroup$
    – Bob Jansen ♦
    Commented May 20, 2015 at 19:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.