Need help interpreting the relationship between interest and loan amount for P2P lending platforms

Question

I'm analyzing the data from a large peer-to-peer lending platform. More precisely, I am analyzing the relationship between the interest rates that are charged by lenders and the loan amount that they grant. I make a distinction between high-risk (bad credit rating) and low-risk (good credit rating) borrowers.

I am doubting whether I should assume a linear relationship between interest and amount (for both high-risk borrowers and low-risk borrowers) or a quadratic relationship. See the pictures below, these are the relationships I find.

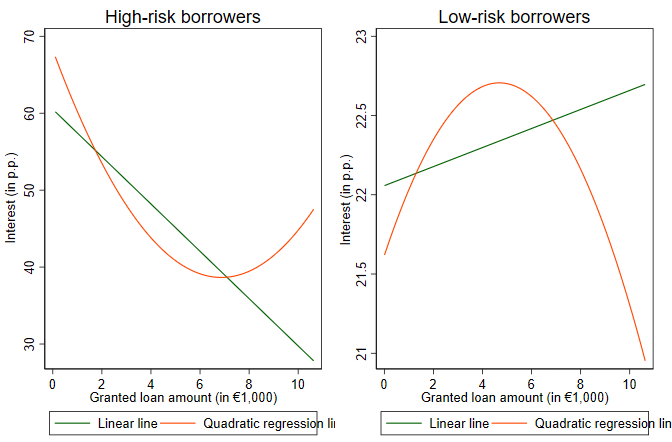
Now does this make sense? The most basic assumption would be that lenders would charge higher interest rates to high-risk borrowers as the probability of default will significantly increase when the granted loan amount increases (i.e. a positive linear relationship between interest and granted loan amount), but this is not the case according to the data. In fact, lenders seem to charge less interest as high-risk borrowers are given larger loans.

What could be a possible explanation for this? My guess is that for high-risk borrowers, lenders seem to compete with other lenders by lowering interest rates as the loan amount increases, as they already charging them (HR borrowers) high amounts of interest. However, at a certain point (around €7,000) they start to charge more interest again, either because (1) the amount of competition decreases and lenders gain more individual bargaining power or (2) the amount of risk that a higher loan bears at this point is so big that lenders want to be compensated by charging more interest.

All in all, I find it kinda difficult to interpret these results. Are there any people here that could offer better explanations?

Answer 1

I don't have enough reputation to write this as a comment, but, in my opinion, looking only at the rate-size relationship is not sufficient as you leave out one really important feature of credit: maturity

In most cases, the longer the maturity of the loan, the higher the compensation asked by the lenders. For example look at yield curves in credit markets. I would highly recommend to include this in your analysis and then look at the results again. Specifically: look at the rate-maturity relationship for high and low rated borrowers. The resulting curves should differ in rates but both increase in duration. Then, on top of that, you can look at loan size for every available term.

Answer 2

Two important things to check

1. Are larger loans going to borrowers with lower risk scores, all else being equal? I would do a multivariate analysis including both loan amount and borrower risk ratings (and term if you have it). It may be that higher loan amounts are going to the lower risk clients.
2. Are the loan terms otherwise the same across loan amounts? Or do you have in fact more than one loan type with higher loan amounts being under terms that would make for lower risk or could compounding be done differently such that actual interest is higher? Are the lower loan amounts more likely to be unsecured, whereas the higher loan amounts are more likely to have collateral?

See also this: https://www.lendingclub.com/foliofn/rateDetail.action

and this: https://www.lendacademy.com/how-much-money-should-you-borrow-at-lending-club/