2
$\begingroup$

I am struggling to understand how was this simple Value-at-Risk calculated. It's Example 1 in Daníelsson, Jón, et al. "Fat tails, VaR and subadditivity." Journal of econometrics 172.2 (2013): 283-291 (a draft of which can be found in this link).

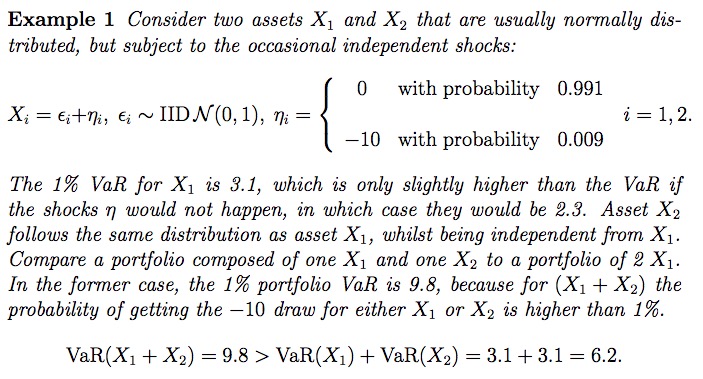
The authors consider a random variable $X_i$ defined as the sum of a standard normal random variable and a discrete random variable (which is independent from the normal r.v.).

I have attempted to obtain the distribution of $X_i$ by considering that it can be seen a mixture of gaussian distributions, with each component of the mixture representing a "regime", but I don't arrive at the same value as the authors (3.1).

I am conscious that this is a very basic exercise, but I must be missing something....

Thank you in advance for any suggestions.

Example 1 in Daníelsson, Jón, et al. "Fat tails, VaR and subadditivity." Journal of econometrics 172.2 (2013): 283-291.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

You can approximate the pdf of the mixture as: $$f_X(x) = 0.009 g(x+10) + 0.991 g(x)$$ for $g(x)$ the pdf of $\mathcal{N}(0,1)$

You will observe that since the standard deviation is 1 almost all of the density attributed to the component $g(x+10)$ is about the point x=-10 and certainly for about 7 standard deviations away about the point x=-3.1 it is effectively all encompassed.

Therefore finding the 1% VaR is equivalent to finding the (1%-0.9%) VaR of g(x), which is in fact -3.09. I suppose the author rounded up.

=NORMINV(0.01-0.009,0,1) gives -3.0902 in excel

edit after comments

If the numbers were not no obviously skewed in this case then you would essentially have the equation:

$$ 0.009 * \Phi(\alpha + 10) + 0.991 * \Phi(\alpha) = 1\% $$ for $\Phi$ the distribution function associated with g(x). Your task is to solve for $\alpha$ for which I don't believe there to be an analytical solution and you would have to use an iterative procedure. The estimation above was essentially 1 iteration since the problem lended itself nicely to the scenario. (And actually note that from this explicit formula you can see I made a mistake by not dividing by 0.991: -3.0902 / 0.991 = -3.118, which is still rounded to -3.1)

Improve this answer
$\endgroup$
3
  • $\begingroup$ This approach is certainly different, thank you. However, I'm failing to see why "finding the 1% VaR is equivalent to finding the (1%-0.9%) VaR of g(x)". I understand that the weight of $g(x+10)$ is 0.009 = 0.9%, but I'm failing to see how is the composition of the mixture $f_X(x)$ summarized in finding the (0.01-0.009) perecentile of $g_X(x)$. $\endgroup$
    – José Luis Molina-Borboa
    Commented Jul 20, 2018 at 6:17
  • $\begingroup$ I think I've got it! If one calculates $\int_{-\infty}^x F_X(x)dx$, when $x$ is well past practically all of the density corresponding to $g(x+10)$ we've "acummulated" 0.009*1, which means we only have to acummulate (0.01-0.009) from the density of $g(x)$. This is an extreme case, because the densities are 10 std. devs. away, how would one approach this problem analitically if the densities were closer together? $\endgroup$
    – José Luis Molina-Borboa
    Commented Jul 20, 2018 at 7:26
  • $\begingroup$ @JoséLuisMolina-Borboa see edits $\endgroup$
    – Attack68 ♦
    Commented Jul 20, 2018 at 7:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.