How to Take Advantage of Arbitrage Opportunity of Two Options

Question

I got the following interview question and corresponding solution, but I have a different understand that might be wrong, so I really appreciate your advice on it:

A European put option on a non-dividend paying stock with strike price 80 dollars is currently priced at 8 dollars and a put option on the same stock with strike price 90 dollars is priced at 9 dollars. Is there an arbitrage opportunity existing in these two options?

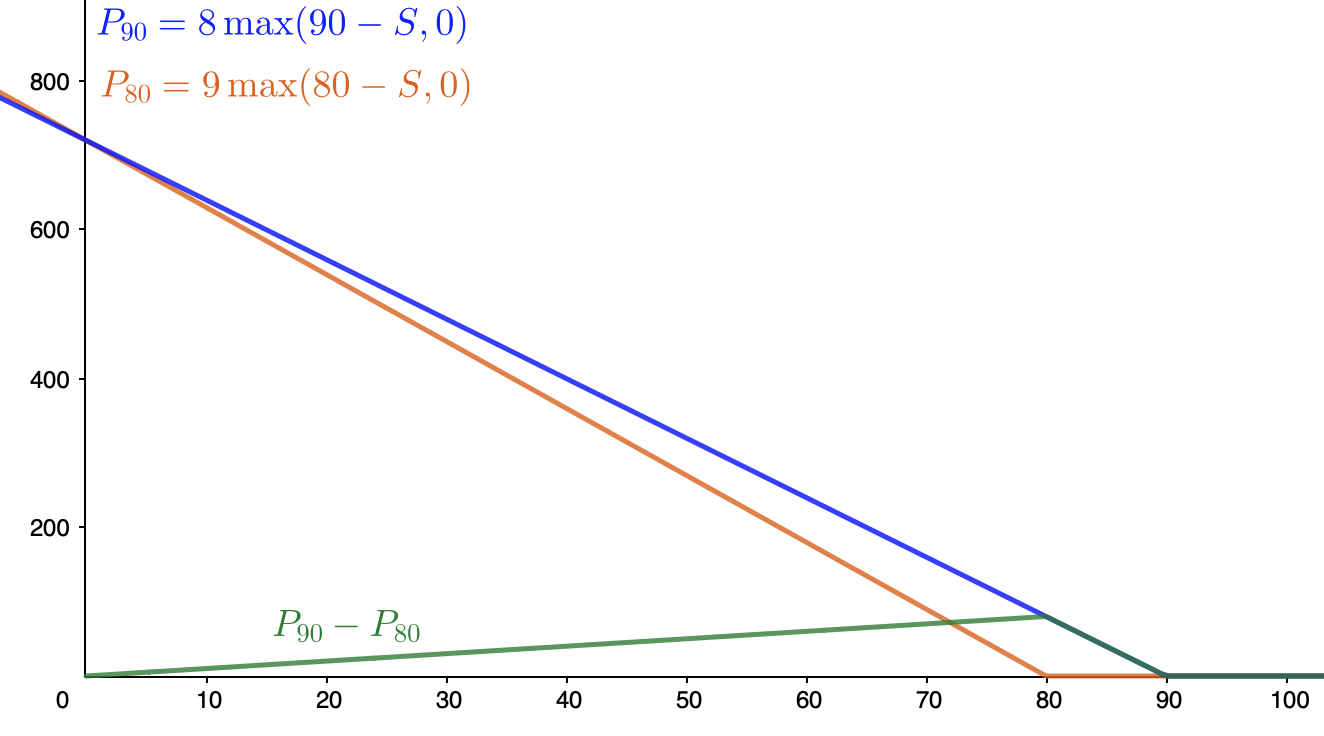
Solution: since the price of a put option as a function of the strike price is a convex function, and since a put option with strike 0 is worthless, we always have $P(0)+aP(K) = aP(K)>P(aK)$. So we have: $(8/9)*P(90) = (8/9)*9 = 8>P(80)$ Since the put option with strike price 80 dollars is currently priced at 8, it is overpriced and we should short it. The overall arbitrage portfolio is to short 9 units of put with $K=80$ and long 8 units of put with $K=90$. At time 0, the initial cash flow is zero. At maturity date, we have three possible scenarios:

$S_T>=90$,payoff=0 (no put is exercised)

$90>S_T>=80$, payoff = $8*(90-S_T)>0$ (puts with K=90 are exercised)

$S_T<80$, payoff = $8*(90-S_T)-9*(80-S_T)>0$ (all puts are exercised)

The final payoff $>=0$ with positive probability. So it is clearly an arbitrage opportunity.

But can I understand the question as follows?

I think the put option with strike 80 is underpriced (instead of overpriced), why? because: by using $aP(K)>P(aK)$ mentioned above, we have:$(9/8)*p(80)>=p[(9/8)*80]=p(90)=8$ So we have $P(80)>=8$, so it is under priced. I'm wondering if I'm wrong?

Answer 1

I think it is far easier to understand by just drawing the payoffs. You have two put options:

• A European put option on a non-dividend paying stock with strike price 80 is priced at 8 dollars, and
• a put option on the same stock with strike price 90 dollars is priced at 9 dollar

The difference between the two payoffs is equal to 10 dollars (90 strike puts payoff exceeds the 80 strike payoff by 10) when both are in the money. Additionally the 90-strike put option pays something in the region between 80 and 90 and the 80-strike put pays nothing in this region. Now two ways to proceed to create arbitrage: 1) zero cost, positive payoff, 2) negative cost, non-negative payoff. let's go with the first:

Buying 8 options of 90-strike will cost 8 times 9=72, selling 9 options of 80-strike will generate the same amount (9 times 8=72). So the cost of the strategy is zero. The payoff diagram is as follows:

PS: And for the convexity logic, if you plot the given option prices as a function of strike, you get a straight line. Convexity would imply the price of 80-strike should be slightly lower?