Clarification on Paul Wilmott's derivation of Ito's Lemma

Question

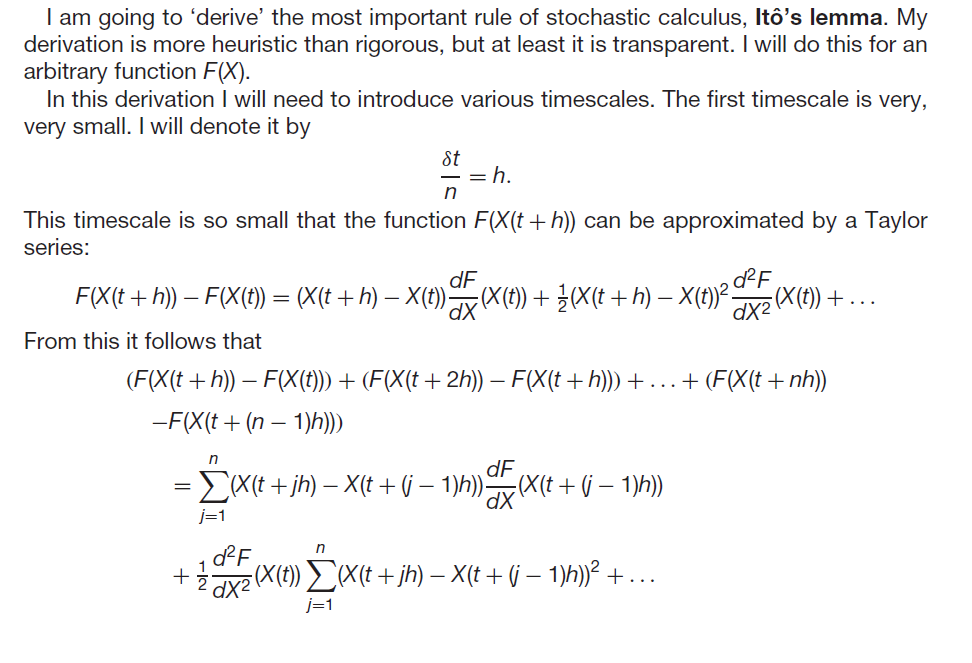
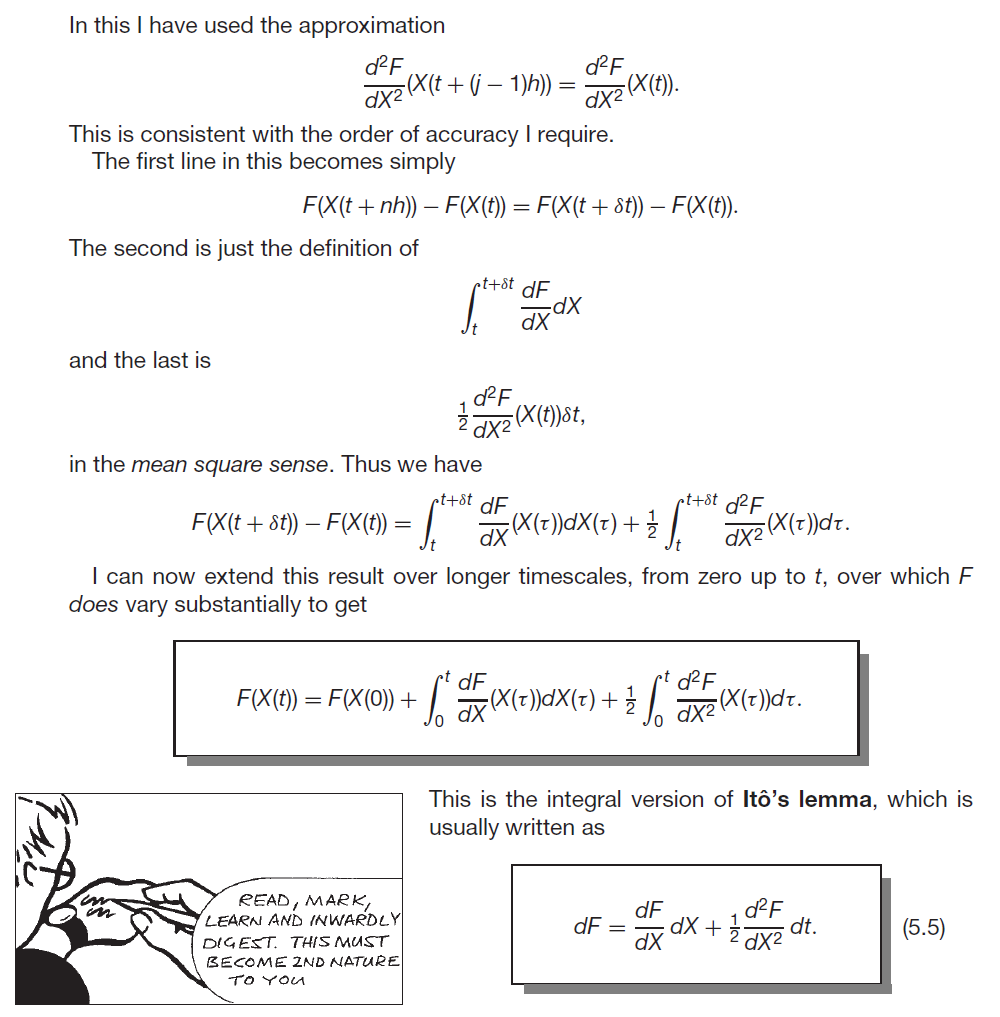
I'm currently self-studying to be quant and have been thoroughly enjoying PW's book. I have some questions regarding his derivation of Ito's lemma. Specifically, I can see that the first line in his expansion comes from

$$ \begin{split} (F(X(t + h)) - F(X(t))) + (F(X(t + 2h)) - F(X(t + h))) + . . . + (F(X(t + nh)) -F(X(t + (n - 1)h)))\\ = F(X(t + nh)) -F(X(t + (n - 1)h)) + F(X(t + (n - 1)h)) - ... - F(X(t + 2h)) + F(X(t + 2h))\\ - F(X(t + h)) + F(X(t + h)) - F(X(t))\\ = F(X(t + nh)) - F(X(t))\\ = F(X(t + \delta t)) - F(X(t)) \end{split} $$ By using the definition $$ \begin{split} W(t) &= \int^t_0 f(\tau)dX(\tau)\\ &= \lim_{n\to\infty}\sum_{j=1}^{n} f(t_{j-1})(X(t_j) - X(t_{j-1}))\\ &= \lim_{n\to\infty}\sum_{j=1}^{n} f((j-1)t/n)(X(jt/n) - X((j-1)t/n))\\ \end{split} $$ which I can see that the second line becomes $$ \begin{split} \sum^n_{j=1}(X(t+jh)-X(t+(j-1)h))\frac{dF(X(t+(j-1)h))}{dX} \\ = \sum^n_{j=1}(X(t+j\delta t/n)-X(t+(j-1)\delta t/n))\frac{dF(X(t+(j-1)\delta t/n)))}{dX}\\ = \int^{t+\delta t}_{t} \frac{dF}{dX}dX \end{split} $$ Finally, what I don't quite see if how the final term goes from $$ \frac{1}{2}\frac{d^2F(X(t))}{dX^2}\sum^n_{j=1}(X(t+jh)-X(t+(j-1)h))^2 $$ to $$ \frac{1}{2}\frac{d^2F}{dX^2}(X(t))\delta t $$ to then become $$ \frac{1}{2}\int^{t+\delta t}_{t}\frac{d^2F(X(\tau))}{dX^2}d\tau $$ If in the mean square limit of $n\rightarrow\infty$ for $t_j = jt/n$, $$ \sum^n_{j=1} (X(t_j)-X(t_{j-1}))^2 = \sum^n_{j=1} (X(jt/n)-X((j-1)t/n))^2 = t $$ then I don't quite see how $$ \sum^n_{j=1} (X(t+jh)-X(t+(j-1)h))^2 = \sum^n_{j=1} (X(t+j\delta t/n)-X(t+(j-1)\delta t/n))^2 = \delta t ? $$ don't they differ by a $t + $ term? Perhaps I don't see this due to my maths being rusty. And also finally, I don't see how $\frac{1}{2}\frac{d^2F(X(t))}{dX^2}\delta t$ becomes $\frac{1}{2}\int^{t+\delta t}_{t}\frac{d^2F(X(\tau))}{dX^2}d\tau$ when the integrand is also dependent on $\tau$.

Answer 1

Given my earlier comment, the only open question is how $\frac{1}{2}\frac{d^2F(X(t))}{dX^2}\delta t$ becomes $\frac{1}{2}\int^{t+\delta t}_{t}\frac{d^2F(X(\tau))}{dX^2}d\tau\,.$ A more standard proof is this: Writing $$ t_j=t+jh $$ we have

\begin{align} &\sum_{j=1}^n\frac{d^2F}{dX^2}\Big(X(t_{j-1})\Big)\Big(X(t_j)-X(t_{j-1})\Big)^2\\ &=\sum_{j=1}^n\frac{d^2F}{dX^2}\Big(X(t_{j-1})\Big)\Big(X^2(t_j)-X^2(t_{j-1})+2X(t_{j-1})(X(t_{j-1})-X(t_j))\Big)\\ &\to\int_t^{t+\delta t}\frac{d^2F}{dX^2}\Big(X(\tau)\Big)\,dX^2(\tau)-2\int_t^{t+\delta t}\frac{d^2F}{dX^2}\Big(X(\tau)\Big)\,X(\tau)\,dX(\tau)\\ &=\int_t^{t+\delta t}\frac{d^2F}{dX^2}(X(\tau))\,d\tau\,. \end{align} The last line follows from $dX^2=2X\,dX+dt\,.$ Wilmott's approximation $$ \frac{d^2F}{dX^2}(X(t_{j-1}))=\frac{d^2F}{dX^2}(X(t)) $$ is not needed.