PD for ECL modelling

Question

I am trying to understand the interrelations between the marginal, cumulative and conditional PDs (Probabilities of Default) when modelling ECLs (Expected Credit Losses). My current understanding is that for a loan with remaining maturity of $t$ years the following calculations apply:

Conditional $PD_{t} = (PD_{t}|PD'_{t-1})$

Marginal $mPD_{t} = PD_{t}*(1-PD_{t<n})$

Cumulative $cPD = \sum_{t=1}^{n} mPD_{t}$

Alternatively $mPD = cPD_{t}-cPD_{t-1}$

A numerical example would be as follows:

$t = 3$

$PD_1 = 5\%$ for simplicity we we assume independenceso that $PD_{t} = PD_{t-1}$, which gives us the following tree diagram: 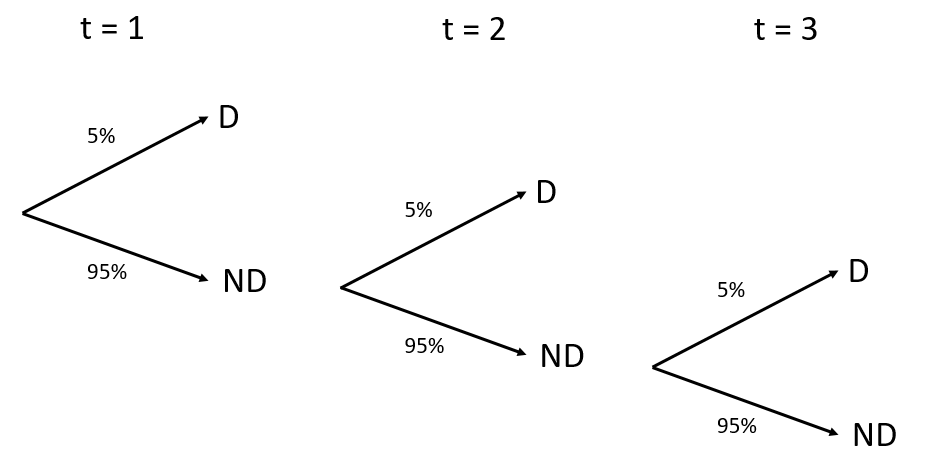From the diagram it follows that:

$mPD_{1}=5\%$

$mPD_{2}=5\%*95\% = 4.75\%$

$mPD_{3}=5\%*95\%*95\% = 4.51\%$

$cPD_{1} = 5\%$

$cPD_{2} = 5\%+4.75\%=9.75\%$

$cPD_{3} = 5\%+4.75\%+4.51\%=14.26\%$

My question is what numbers should be applied when modelling ECLs. For example if we have a 3 year loan for 1m USD at 5% interest (for simplicity lets assume that the payments are made annually) then the annual payments will be 367,208 USD. So if we want to calculate the ECLs for this loan do we need to apply the marginal or conditional probabilities? And to what to we apply these probabilities to, the cash flows or the remaining balance of the loan?

Answer 1

All that you have highlighted is well posed.

It makes sense to use conditional PD values applied to the remaining balance.

In fact, the expected loss is indeed a measure used to understand how much exposure one has to the default of the counterparty. Therefore, if a counterparty has paid back a part of the debt, then the only default risk exposure is on the stake not paid yet, i.e. the outstanding debt.

In general, you should differentiate two approaches in credit risk: default risk and mark-to-market risk. The first is solely the probability of default, the second takes also into consideration the changes in the credit quality of the counterparty, which are well described by rating changes. That is why you should use conditional PDs. In the case of calculating the EL - which is a default measure - you want to consider the PD coming from a filtration (i.e. you want to take into account that the PD may change in response to changes of various factors affecting the PD - systematic factors, as well as idiosincratic ones), therefore makes sense to look at conditional PDs.

When it comes to Credit risk, I like this paper: https://www.researchgate.net/publication/247333419_Ratings_migration_and_the_business_cycle