How to hedge a bull call spread

Question

I am trying to make a theoretical hedge to a bull call spread. (buy out the money call, sell further out the money call)

What I have now is almost effective but there is one possible 80% loss (amongst consistent 70% gains in an equally likely scenario, and 300% gains in an extreme scenario)

Best case scenario: 70% gain

Worst case scenario: 80% loss

Black swan bearish scenario: 300+% gain (this is a factor of the hedge)

What I would like my hedge to do is mitigate the worst case scenario.

Here is the rationale: QQQ Bull Call Spread in near term, this is bullish (qqq represents the nasdaq composite)

FAZ long calls in back month (to mitigate theta), this is bearish as FAZ is a 3x leveraged ETF (albiet on the finance sector). FAZ will increase in value 3x for every 1 point move down QQQ makes. Calls will get intrinsic value very quickly.

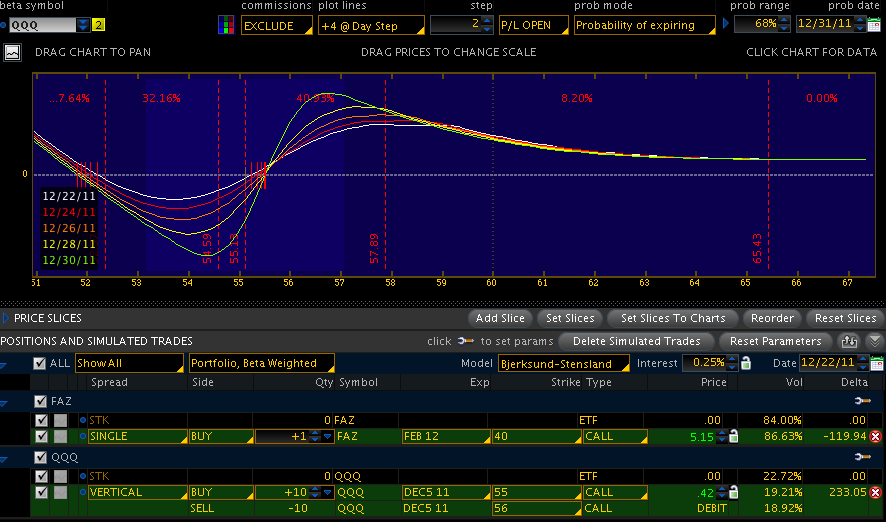
For this site's sake, I'm not caring too much about the symbols. I am interested trying to find a cheap hedge that increases 3x faster if the other side of the trade fails. Right now I almost have that, but not yet.

The key variables to manipulate are:

Balance: How much of the hedge is held in proportion to the main trade. This simulation shows 10 bull call spreads, hedged by 1 long call in an inverse ETF

Theta: The front month expires faster than the back month. The back month hedge can be closed before the effects of theta become apparent. But the further out you go for the back month, the most expensive it gets

Expense: the hedge ideally should not be more expensive than the potential profit of the main trade, but it is expected to cut into the theoretical max profit of the main trade.

The key is to get the shape of the risk profile to have a smaller dip into the negative at any point on the graph.

Answer 1

I suggest searching all the possibilities using Excel. Code the option pricing formulas into VBA functions (if you have not done so already) with cell to hold option strikes and quantities, and then set up your price scenarios for a grid of 1% moves in the underlying. You have now reproduced the information contained in your Bloomberg plots pictured above.

Excel Solver will let you do a search on the outcomes, and even set constraints such as never losing more than 40% (as opposed to the 80% you now have). You will want to define a particular "utility function" cell for it to maximize, perhaps the peak profit or whatever else fits your personal desiderata. Start the solver with the best scenario you have established so far, and let it improve your utility function within whatever constraints you have set.

Obviously some hypothetical constraints will be impossible to satisfy, e.g. 0% downside and >0% upside in all market scenarios.