How might I answer this past exam question relating to the limiting price of an option?

Question

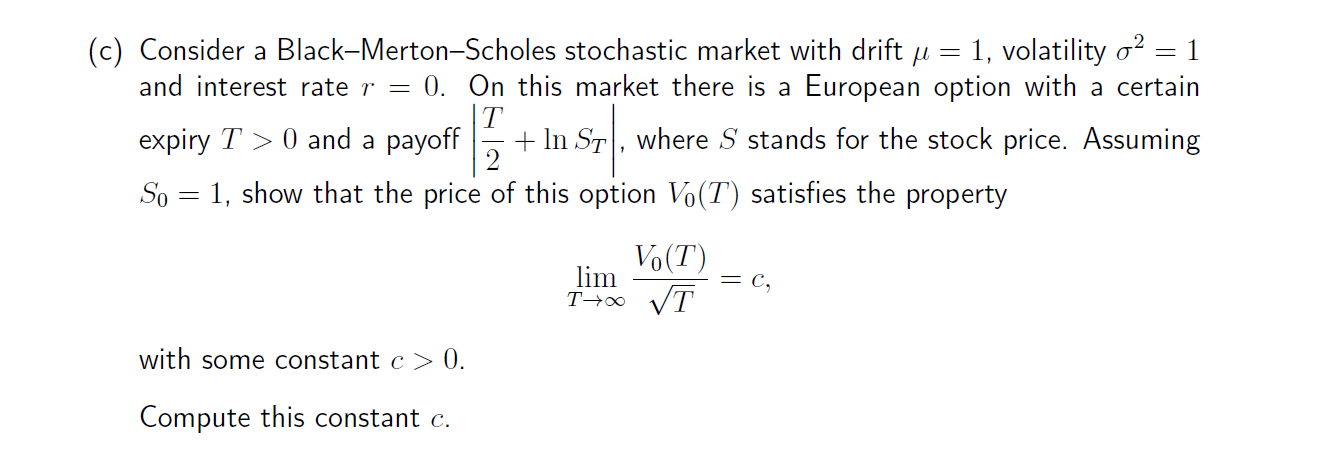
The following image shows a past exam question that I am attempting to answer (for which I do not have a mark scheme):

I believe that under the BMS model, the payoff of a stock at maturity $T$ is given by $$ S_T = S_0 \exp \left( \mu T + \sigma W_T \right) $$

Thus, the payoff of the stock in the question would be given by $$ S_T = \exp \left( T + W_T \right) $$

Therefore, I would expect the payoff of the option to be $$ V_0 (T) = \left| \frac{T}{2} + T + W_T \right| = \left| \frac{3T}{2} + W_T \right| $$

However, $$ \lim_{T \rightarrow \infty} \frac{\left| \frac{3T}{2} + W_T \right|}{\sqrt{T}} = \lim_{T \rightarrow \infty} \left| \frac{3\sqrt{T}}{2} + \frac{W_T}{\sqrt{T}} \right| = \infty $$

What am I doing wrong?

Answer 1

Two things:

• The price of the option is the expectation under the risk-neutral measure. Under this measure, the stock price's drift is $r$ and not $\mu$: $$dS_t = S_t r dt + S_t \sigma dW_t$$

• When you integrate to get $S_T$, you have made a mistake: $$S_T = S_0\exp\left(\left(r-\frac{\sigma^2}{2} \right)T + \sigma W_T \right)$$

Start by writing out the expression of $d\ln(S_t)$ using Itô's lemma, then deduce $\ln(S_T)$.