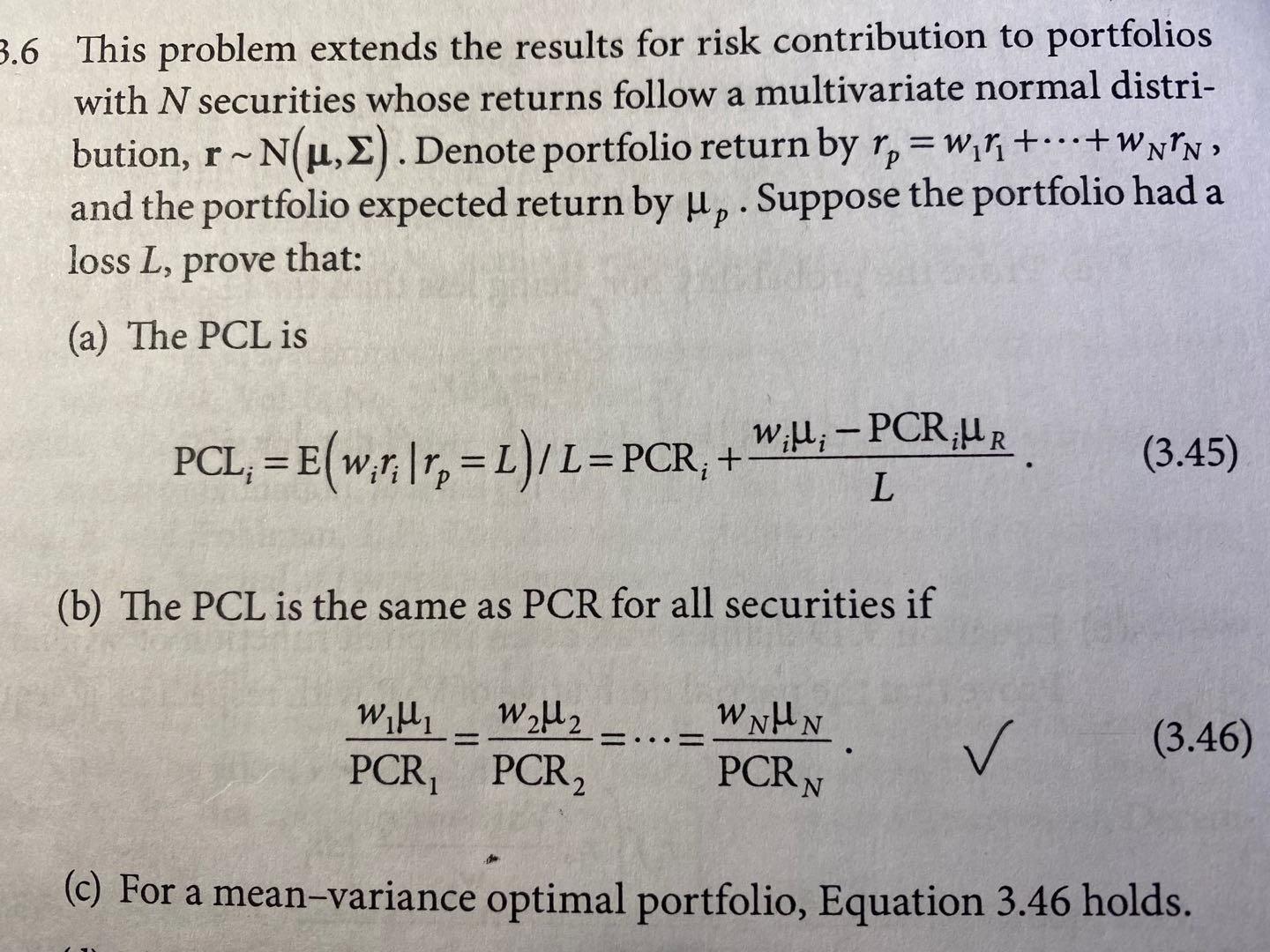
I came across this question during self study on a quantitative book (Question 3.6 on Page 75 of Quantitative Equity Portfolio Management: Modern Techniques and Applications By Edward E. Qian, Ronald H. Hua, Eric H. Sorensen ), can someone help me out? I got stuck on part(c). FYI, PCR = Percentage Contribution to Risk, PCL = Percentage Contribution to Loss, the definition for PCR is: $$PCR_i=\frac{\omega_i\frac{\partial\sigma}{\partial w_i}}{\sigma}$$ and I have already found the optimal $\displaystyle \omega^*$ and optimal $\displaystyle (\sigma^*)^2$ from mean-variance optimization problem formed as follows. $$Maximize \ \ \ \ \ \ \omega^T\cdot f-\frac{1}{2}\lambda(\omega^T\Sigma\omega )\\ subject \ \ to \ \ \ \ \ \ \omega ^T i=1$$
$\begingroup$
$\endgroup$
8
-
$\begingroup$ What is $f$ in your objective function $\endgroup$– develaristCommented Oct 14, 2020 at 16:45
-
$\begingroup$ It seems like just a multivariate extension of what they have in the main text, where they use the conditional expectation of one normal random variable given the sum. The only novelty seems to be $\mu_R$ which might refer to $\mu_P$. $\endgroup$– Magic is in the chainCommented Oct 14, 2020 at 19:49
-
$\begingroup$ @develarist f is a input variable which stands for forecast return $\endgroup$– tbzjCommented Oct 15, 2020 at 0:56
-
$\begingroup$ expected (average) return $\mu$? And what is $\sigma$ in the PCR formula? portfolio $\sigma_p$ or asset $\sigma_i$? $\endgroup$– develaristCommented Oct 15, 2020 at 1:22
-
$\begingroup$ @develarist $f$ is just the return for each stock, we can obtain expected return for the optimal portfolio by multiplying $f$ and $\omega^*$. Also $\sigma$ is total risk of the portfolio which is $\sigma_p$ $\endgroup$– tbzjCommented Oct 15, 2020 at 3:01
|
Show 3 more comments