Pareto comparison of return distributions

Question

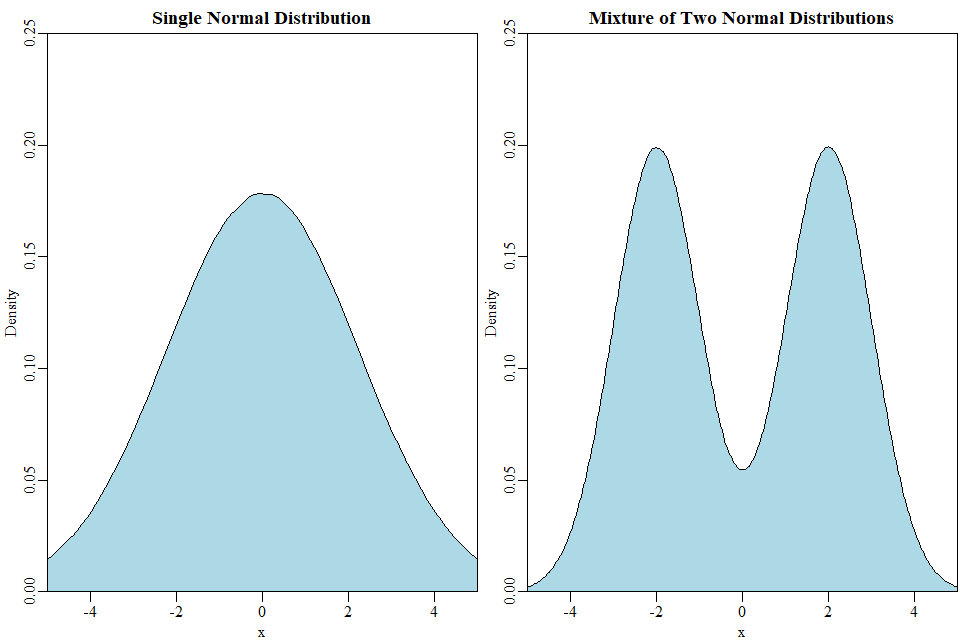
In making a choice among financial strategies, each of which has some estimated return distribution, some strategies will clearly be better than others. But many times, the choice is a question of risk tolerance. That said, there's still a problem: the risk/reward tradeoff is often too coarse -- because different distributions (e.g., outputs from a monte carlo simulation) may have the same mean and standard deviation while being qualitatively different (e.g., see image below).

Is there any more general, principled way to compare arbitrary probability distributions of expected returns? Like defining some kind of (maybe multi-dimensional) pareto curve over PDF space? Or another quantitative technique to make this selection process more rigorously grounded?

Given a set of modeled strategies, and return distributions like these ones, how can we identify the set of "dominating" distributions that are "best" in some sense? How can we rigorously guide the selection among them?

Answer 1

To expand a bit on my comment: The idea of stochastic dominance may help you here.

Comparing two distributions $A$ and $B$, we say that $A$ stochastically dominates $B$ ($A\succcurlyeq B$)if certain conditions (to be made preice) regarding the distributions hold. The nice thing is that these conditions relate to general aspects of preferences and utility, without specifying a fixed utility function.

So for example, distribution $A$ first order stochastically dominates $B$, ,$A\succcurlyeq_{\mathrm{1st.\ ord.}} B$ if for any outcome $x$, the probability of reaching $x$ or more is equal or higher under $A$ than under $B$ (with at least one strict inequality). This is the case

if and only if every expected utility maximizer with an increasing utility function prefers gamble A over gamble B

For second-order stochastic dominance, $A$ will dominate $B$ if and only if

$E[u(A)]\geq E[u(B)]$ for all nondecreasing and concave utility functions $u(x)$.

Conveniently, this directly translates to the condition

$$ \int_{-\infty}^x[F_B(t)-F_A(t)]\mathrm{d}t\geq 0 $$ which is easily calculated.