I am looking for help to derive this formula from Brigo & Mercurio

Question

I think my use of numeraire change is incorrect and for sure my understanding is incomplete. $\frac {dQ2}{dQ1}=\frac {Pt(0,T2)P0(0,T1)}{P0(0,T2)Pt(0,T1)} = \frac {1+DeltaF_1(t)}{1+DeltaF_2(t)}$

Then by Radon–Nikodym $E^{Q2}[F2(t)]=E^{Q1}[F2(t)*\frac {dQ2}{dQ1}]=E^{Q1}[F2(t)*\frac {1+DeltaF_1(t)}{1+DeltaF_2(t)}]$ also by Ito I know that $ F_2(t) = F_2(0) \exp\left( -\frac{v_2^2}{2} t + v_2 W_t \right)$

I am stuck from there, I am looking to obtain the last equation and to understand all the steps. I feel like I am taking the wrong path or I misunderstood the numeraire change technique or girsanov...

Answer 1

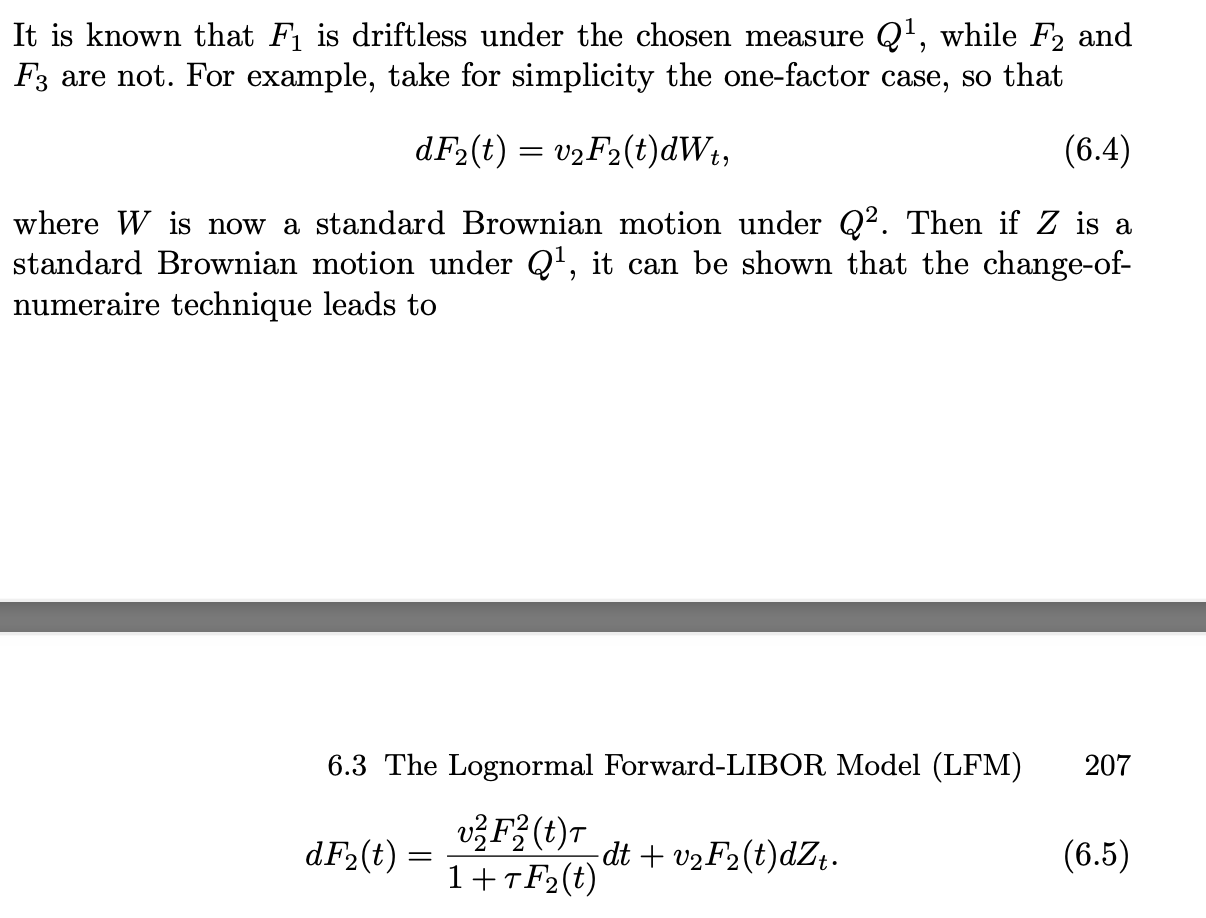
Ok my bad,

So I have $1+\delta L(0,T1,T2)=\frac{P(0,T1)}{P(0,T2)} \tag{1}$

and

$\frac{dL(0,T1,T2)}{L(0,T1,T2)}=v_2dWt $

that gives

$L(0,T1,T2)P(0,T2)=\frac{1}{\delta}(P(0,T1)-P(0,T2)) \tag{2}$

which is a tradable asset.

Then we that if L(0,T1,T2)P(0,T2) is a tradable asset then for any numeraire X it exists a measure where:

$\frac {L(0,T1,T2)P(0,T2)}{X(0)}=E^{X}(\frac {L(s,T1,T2)P(s,T2)}{X(s)} \backslash Ft)$ ie the asset is martingale. From there and according to $(2)$ we can easily see that the rate $L(0,T1,T2)$ is a martingale under $Q2$. Now lets calculate our drift under $Q1$ measure:

$\frac {L(0,T1,T2)P(0,T2)}{P(0,T1)}=E^{Q1}(\frac {L(s,T1,T2)P(s,T2)}{P(S,T1)} \backslash Ft)$

Which gives us

$L(0,T1,T2)=E^{Q1}(\frac {L(s,T1,T2)P(t,T2)P(0,T1)}{P(t,T1)P(0,T2)} \backslash Ft)$

we then have the radon Nikodym derivative $\frac {dQ2}{dQ1}=\frac {P(t,T2)P(0,T1)}{P(t,T1)P(0,T2)}$

$(1)$ allows us to rewrite the previous equation $Z=\frac {dQ1}{dQ2}=\frac {1+\delta L(t,T1,T2)}{1+\delta L(0,T1,T2)}$

We can easily see that $dZ=\frac {v_2L(t,T1,T2)dWt}{1+\delta L(0,T1,T2)}$

Then $\frac {dZ}{Z}=\frac {v_2L(t,T1,T2)dWt}{1+\delta L(0,T1,T2)}\frac {1+\delta L(0,T1,T2)}{1+\delta L(t,T1,T2)}=\frac {v_2L(t,T1,T2)}{1+\delta L(t,T1,T2)}dW_2t$

$\frac {dZ}{Z}$ and the fact that $L(0,T1,T2)$ have a log normal dynamic allow us to show directly induce the new Brownian motion from Girsanov theorem:

$dW_1t=dW_2t-\frac {v_2L(t,T1,T2)}{1+\delta L(t,T1,T2)}dt$

so we have

$\frac{dL(0,T1,T2)}{L(0,T1,T2)}=v_2dW_2t=v_2(dW_1t +\frac {v_2L(t,T1,T2)}{1+\delta L(t,T1,T2)}dt)$

Done.