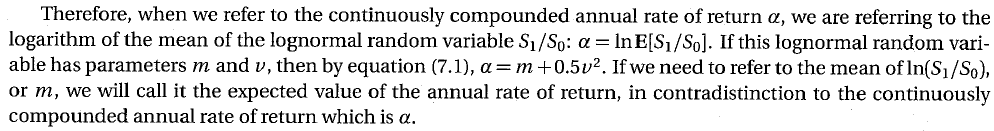Clarification on this author's solution for this problem on lognormal stock distribution

Question

I am self-studying from a manual on financial economics, and I am trying to completely wrap my head around this solution:

I'm trying to fill in the in-between steps of this solution based on first principles, so please tell me if my understanding is correct (I'll be referencing the paragraph from the textbook shown below my solution):

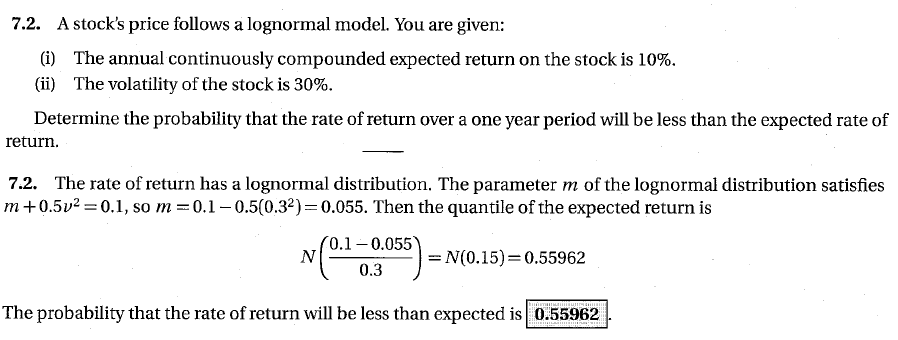
The rate of return over a one year period is $S_1/S_0$. The expected rate of return is $\textbf{E}[S_1/S_0].$

The problem is therefore asking to find $\text{Pr}[S_1/S_0 < \textbf{E}[S_1/S_0]]$.

Now $S_1/S_0$ is lognormally distributed, so we have:

$\text{Pr}[\ln(S_1/S_0) < \ln(\textbf{E}[S_1/S_0])] = \text{Pr}[\ln(S_1/S_0) < \alpha]$.

We have that $\ln(S_1/S_0)$ is normally distributed with parameters $m = 0.1 - 0.5(0.30)^2 = 0.055$ and $v = 0.30$.

Hence $\text{Pr}[\ln(S_1/S_0) < \alpha] = \text{Pr}[z < (\alpha - m)/v] = N(\frac{0.1 - 0.055}{0.3}) = N(0.15) = 0.55962$.

My question is:

1. Is the logic of the in-between steps correct? Please correct any detail that I don't have quite right.

2. What does the author mean by the quantile of the expected return, and why is that helpful?

Answer 1

1. Yes, your steps are valid
2. This is a wrong use of the term "quantile". Here you need to compute a probability (through the normal cdf) and not a quantile (i.e. the value of a random variable corresponding to a given level of the cdf, e.g. the quantile 0.5 (or percentile 50%) is the median)