Why the interest rate for put-call parity is not constant?

Question

Usimg the put-call parity

$C - P = S - K · e^{-rt}$

I tried to estimate the value of $e^{-rt}$, the present value of a zero-coupon bond that matures to 1 in time $t$:

$e^{-rt} = (P - C + S) / K$

Where $C$ and $P$ are call and put prices, respectively, calculated as the average between bid and ask prices; $S$ is the price of the underlying and $K$ is the strike price.

I tried this with the SPX options with expiration Dec 2013.

I expected to get a constant $e^{-rt}$, but I got a decreasing $e^{-rt}$ instead. Why is this?

Edit: The spreadsheet that generated the chart

Answer 1

To answer a question with a question - are you assuming proportional or constant dividends? :)

The general consensus of the market is that dividends are somewhere between proportional (fixed yield) and constant (fixed dollar). The carry embedded into the forward prices at different strikes reflects that consensus, in fact you can establish the "constantness" of the dividends based on the slope of the curve (and you will see that it's mostly constant).

Mathematically, you should think of it this way. In a fixed yield dividend model, you have $F_t = S_0e^{t(r-y)}$ where $y$ is your dividend yield. Conversely, for a fixed dollar dividend model, your you have $F_t = S_0e^{tr} - D$ where $D$ is your constant dollar dividend. If you assume the second is true in the market, inverting the first one will give you different dividend yields for different levels of forwards, with dividend yield decreasing as your forward strike is increasing.

Answer 2

Dividends are the key.

For simplicity, let's include a single dividend at the time of expiration, and assume that the options are European and expire ex. (There is really no reason not to assume that an option on a market index is European. EDIT: not quite true; that's discussed here.)

$S+P = e^{-rt}K+C + e^{-rt}D$

This is a certain fixed dividend, but that is not material to our purposes. Whether or not the dividend is subject to market risk and to what degree, or whether or not the dividend is correlated with a change in price of the underlying, it doesn't matter, because in any event, the present value of dividend(s), or what the market expects it to be, has absolutely nothing to do with the strike price of an option.

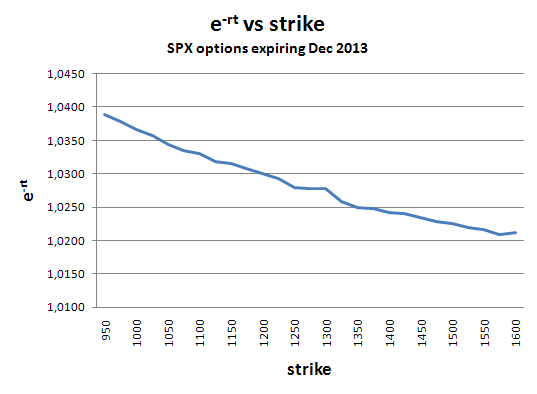
All is not lost:

$e^{-rt} = \dfrac{S+P-C}{K+D}$

Take your spreadsheet, and solve for a fixed $D$ that makes that line flat. Then you will have $e^{-rt}<1$ as expected, and $e^{-rt}D$ is the present value the market places on underlying dividends from now to expiration.

EDIT #2 alternate way to work this out:

$S+P_1 = e^{-rt}K_1+C_1 + e^{-rt}D; \\ S+P_2 = e^{-rt}K_2+C_2 + e^{-rt}D.$

Subtract the first equation from the second:

$P_2-P_1 = e^{-rt}(K_2-K_1) + C_2 - C_1.$

Rearranging terms

$C_1-C_2+P_2-P_1 = e^{-rt}(K_2-K_1)$

we have created a synthetic bond from options at two different strikes which has an unambiguous interest rate because the value of any underlying dividends has cancelled out.

Answer 3

In the Put-Call parity you assume that a risk-free rate $r$ exists, but that's not the case in reality; there is no using risk-free rate. But I can't tell you why it's decreasing, but it's not surprising that it's not constant.

What's more surprising though, is that $e^{-rt}>1$ is should be smaller. Could you plot $r$ only?

Answer 4

In the Put-Call parity r is assumed to be risk-free interest rate.

In reality, the interest rate the is rate at which interest is paid by a borrower for the use of money that they borrow from a lender. Its behavior is similar to price in the market , which price fluctuation depends on the news in the market. It is usually higher than risk-free interest rate. because of the consideration of inflation mainly controlled by money supply and risk-premium that the lenders may not receive the money returned from the borrowers.