4
$\begingroup$

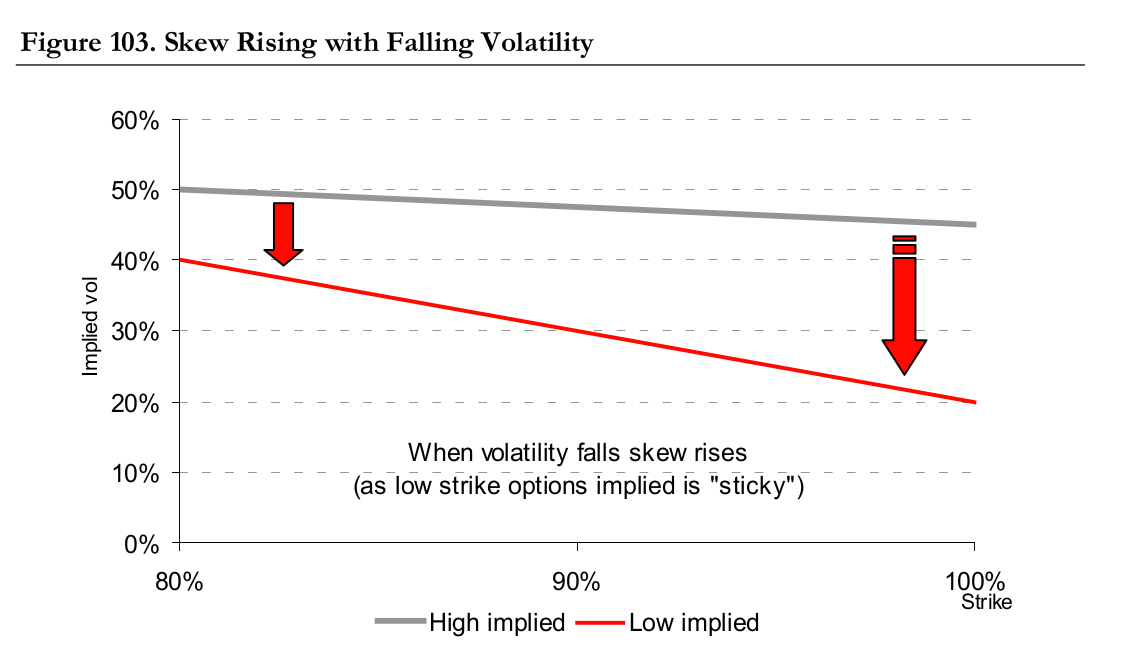
In Trading Volatility by Bennett, he says:

If there is a sudden decline in equity markets, it is reasonable to assume realised volatility will jump to a level in line with the peak of realised volatility. Therefore, low-strike, near-dated implieds should be relatively constant (as they should trade near the all-time highs of realised volatility). If a low-strike implied is constant, the difference between a low-strike implied and ATM implied increases as ATM implieds falls. This means near-dated skew should rise if near-dated ATM implieds decline (see Figure 103 above).

Doesn't this imply that there is a positive spot-skew correlation? When equities fall, we expect the low-strike implieds to remain relatively constant. However, ATM implied volatility usually goes up when equities fall. Therefore, the difference between low-strike implies and ATM implies will decrease as ATM implies rises (as it does when equities decline).

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

The description by Bennett is not very clear, but the reference to the Figure 103 in the text at the end of the paragraph that you cite should resolve the issue.

Bennett is saying that once the sudden equity decline has happened and volatility falls subsequently, ATM implieds fall first with low-strike implieds being sticky. In the figure he is describing the situation when volatility falls, not when volatility rises initially.

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ When you say "once the sudden equity decline has happened and volatility falls subsequently", by "subsequently", do you mean like "after vol first goes up (as it does when equities decline), and then after a while when it comes back down..."? $\endgroup$
    – Jerry Quin
    Mar 17, 2023 at 16:17
  • $\begingroup$ Correct. He is using the example of a sudden equity decline, when volatility would settle subsequently. $\endgroup$
    – Hans-Peter Schrei
    Mar 17, 2023 at 16:30
  • 1
    $\begingroup$ He is using the example of a sudden equity decline [in 1 day, with volatility concomitantly rising], [and] volatility would settle subsequently [slowly, in the following days and weeks] $\endgroup$
    – nbbo2
    Mar 18, 2023 at 6:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.