4
$\begingroup$

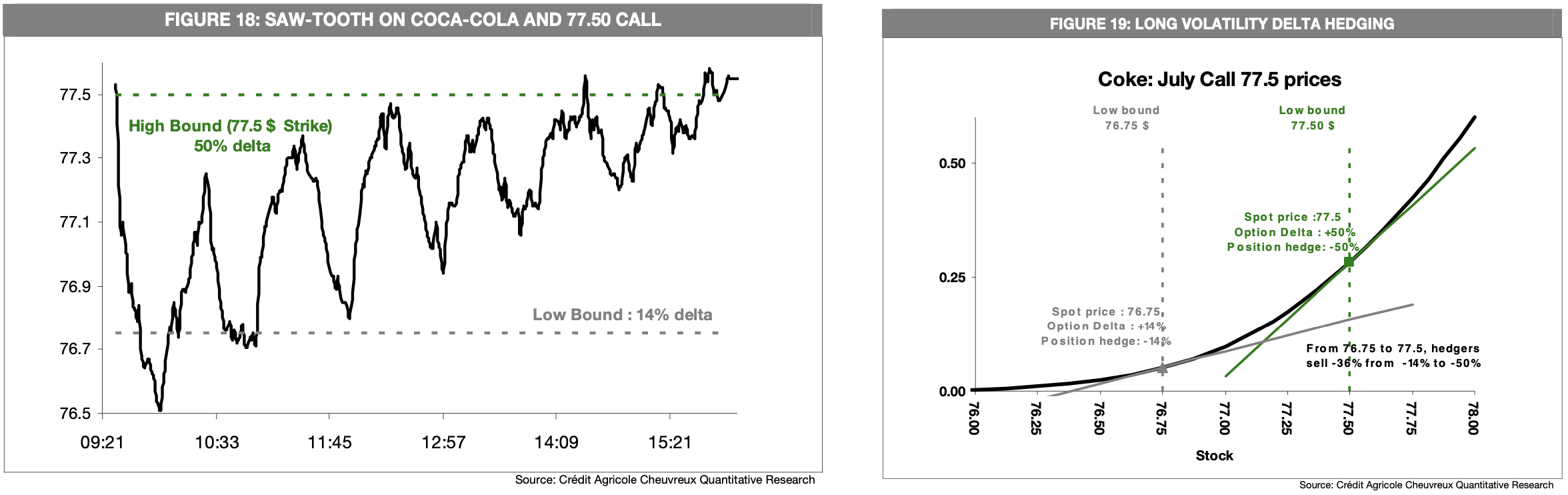
Since options contracts are created by open interest in the contract, it is conceivable that the notional of the total options contracts can exceed the value of the underlying. If that happens, does the underlying become the derivative of the options?

This is more than just a theoretical question. I have started to see in some markets with large options open interest where the delta hedging of the options contracts start to impact the volatility of the underlying--particularly in high gamma/convexity contracts. Those that have negative gamma end up having to buy the underlying in large up moves and exacerbate the volatility on the upside. Conversely those with positive gamma do the opposite in large down moves. In these markets, would we see larger smiles?

Have there been any studies of this feedback phenomenon? Any literature recommendations would be appreciated.

Improve this question
$\endgroup$
5
  • 4
    $\begingroup$ I don't think a systematic study has been carried out because these things have not occurred often enough. The only one I remember is the Porsche Volkswagen incident. I dont think the underlying becomes the option as there may not be a well defined inverse mapping from option(s) to underlying. $\endgroup$
    – user34971
    Commented Apr 13, 2022 at 15:53
  • 1
    $\begingroup$ Secondary market effects do not mean that "the underlying becomes a derivative of the derivative" in the literal sense, but I don't think that's your real question. $\endgroup$
    – D Stanley
    Commented Apr 14, 2022 at 2:04
  • $\begingroup$ @DStanley Yes, I guess what I am really asking is when does the derivative cause volatility rather than just using it as the input in pricing the option (ie pricing volatility)? Is the tail wagging the dog? $\endgroup$
    – AlRacoon
    Commented Apr 14, 2022 at 4:34
  • 1
    $\begingroup$ there are examples in the CDS market where CDS prices drive underlying bond prices rather than the opposite (see p33 of this reference) iosco.org/library/pubdocs/pdf/IOSCOPD385.pdf $\endgroup$
    – dm63
    Commented Apr 15, 2022 at 5:00
  • 1
    $\begingroup$ If the related (european style) options market is significantly more liquid than the reference asset, one could resort to delta hedging via put-call combos. Stock options might not hold as American $\endgroup$
    – James Spencer-Lavan
    Commented Apr 15, 2022 at 8:40

1 Answer 1

Reset to default
4
$\begingroup$

To illustrate his paper on optimal trading for options, Robert Almgren issued this nice pdf with interesting feedback effects.

He reproduces this graphs from What does the saw-tooth pattern on US markets on 19 July 2012 tell us about the price formation process by L et al.:

feedback effect on coke

This interesting effect has to be complemented with pinning as illustrated by A market-induced mechanism for stock pinning, by Avellaneda and Lipkin in 2003. Pinning is more "price manipulation" (when it can be interesting for big participants to push the price above or below the strike at expiry) and nowadays regulators are looking carefully at it.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks very much for your thoughtful answer and the reference. And yes pin risk is real and something traders should be aware of, particularly close to expiry. $\endgroup$
    – AlRacoon
    Commented Apr 15, 2022 at 16:49

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.